This is an archive copy of the IUCr web site dating from 2008. For current content please visit https://www.iucr.org.

Introduction

Most monographs on physics discussing the physical properties of matter usually proceed from isotropic materials and as a generalization include a more or less limited description of the behaviour of crystalline bodies. This way of presentation is doubtless advantageous, however, it implies a separate discussion of the various properties, inevitably obscuring the general principles and methods applicable in the theory of crystal properties. The purpose of the present work is to discuss and summarize in a unified treatment the physical properties of crystals, and to illustrate by some typical examples the principles and methods underlying a uniform description of these properties.

For an introduction, in order to develop a clear crystal physical picture, let us investigate the problem of the dielectric susceptibility in an isotropic and an anisotropic medium respectively.

 

Figure 1: Formation of dipoles (a) in isotropic, (b) in anisotropic insulators.

In isotropic insulators the dipoles generated in the course of dielectric polarization are parallel with the electric field (Fig. 1a), consequently the relation between the vector of the electric polarization ($\=P$) and the electric field ($\=E$), that is the susceptibility, can be characterized by one single value ($\chi$).

$$\=P=\chi\=E.$$ (1.1)

In an anisotropic medium, however, the dipoles formed during the dielectric polarization are generally not parallel with the electric field. Fig. 1b depicts the relatively simple case in which the dipoles though arranged in a plane parallel with the electric field (represented by the plane of the drawing) have a different direction. In this case the vector of the electric polarization ($\=P$) due to the vertical electric field ($\=E$ = $\=E_v$)) has not only a vertical ($\=P_v$) but also a horizontal component ($\=P_h$). Consequently in the case of Fig. 1b two quantities ($\chi_{vv}$; $\chi_{hv}$)are necessary to describe the relation between the vertical electric field and the electric polarization, and the components of the two vectors in question are connected by the equations

$$\=P_v = \chi_{vv}\=E_v$$ (1.2)

$$\=P_h = \chi_{hv}\=E_v.$$

Generally, when investigating the dielectric polarization in the three dimensional space one finds that, disregarding the higher order effects, in an x₁, x₂, x₃ coordinate system the following equations hold between the components of the vector of the electric field ($\=E$ = [E₁, E₂, E₃]) and the components of the electric polarization ($\=P$ = [P₁, P₂, P₃]):

$$P_1 = \chi_{11}E_1 + \chi_{12}E_2 + \chi_{13}E_3$$

$$P_2 = \chi_{21}E_1 + \chi_{22}E_2 + \chi_{23}E_3$$ (1.3)

$$P_3 = \chi_{31}E_1 + \chi_{32}E_2 + \chi_{33}E_3.$$

This means that altogether nine data are necessary to describe the relation between the electric field and the electric polarization. By means of vector algebra it can be shown that in case of an orthogonal coordinate transformation the nine coefficients in the eq. (1.3) transform as the products of the components of two vectors, i.e. they are components of a second-rank tensor. Consequently the dielectric susceptibility in an anisotropic medium can be described by a second-rank tensor.

Equations (1.3) can be rewritten in an abbreviated form

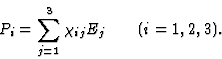$$P_i = \sum^3_{j=1} {\chi}_{ij}E_j \qquad (i = 1, 2, 3).$$ (1.4)

With Einstein's notation the $\sum$ symbol can be omitted if in the same term a suffix occurs twice. Accordingly eq. (1.4) takes the following form

$$P_i = \chi_{ij}E_j \qquad (i, j = 1, 2, 3).$$ (1.5)

In the forthcoming discussions the Einstein convention will be used.

Copyright © 1984, 1998 International Union of Crystallography