Next: 8. Curie's Principle Up: An Introduction to Crystal Physics (Description Previous: 6. Higher Order Effects
![[IUCr Home Page]](/iucr-top/logos/iucrhome.gif)
![[Commission Home Page]](/iucr-top/logos/cteach.gif)
![]()
![]()
![]()
Next: 8. Curie's Principle
Up: An Introduction to Crystal Physics (Description
Previous: 6. Higher Order Effects
It has been shown in the previous sections that the various physical properties of the crystals can be described quite suitably with tensors. However, especially with higher rank tensors the great number of suffixes may become inconvenient. In many cases the introduction of a new notation, the matrix notation, is suitable to reduce the number of suffixes. The introduction of the matrix notation may be encouraged by the usually considerable reduction, due to intrinsic symmetries, of the number of independent components of the tensors representing the physical properties.
Let us consider first a simple example. For some purpose the following
(![]() ) matrices are formed from the components of the symmetrical second
rank [
) matrices are formed from the components of the symmetrical second
rank [![]() ] tensor
] tensor
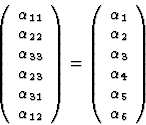 |
(7.1) |
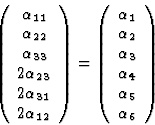 |
(7.2) |
| (7.3) |
This way by introducing the matrix notation the number of the suffixes is reduced on the condition that the new suffixes beside 1, 2 and 3 can now take also the values 4, 5 and 6. Although the relationship between the suffixes of the tensor components and matrix elements is unambiguous, the relationship between the tensor components and the matrix elements is defined to the extent of a multiplication factor. A few examples explaining the role of this factor are given below.
The advantage of the matrix notation becomes obvious with the third and higher rank tensors. A third rank tensor, occurring rather frequently in crystal physics, is the piezo-electric tensor, which describes the relationship between the stresses effective on the crystal and the resulting electric polarization according to the equation
| (7.4) |
| (7.5) |
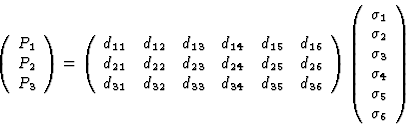 |
(7.6) |
| (7.7) |
It is important to notice that some authors do not introduce the multiplication factor two in the interpretation of the n = 4, 5 and 6 elements of the piezoelectric matrix. This, however, excludes the compact description of the relationship between the vector of the electric polarization and the mechanical stress presented in eq. (7.7).
Similarly other matrices representing various physical properties may be defined by different authors in different ways. Therefore when trying to use the numerical values as published in the literature the various definitions used by the authors should be taken into account.
Finally in order to demonstrate the advantage of the matrix notation let us investigate the elasticity of crystals. Hooke's law takes in tensor notation the following form
| (7.8) |
Now, since the components of the [cijkl] tensor are symmetrical with regard to the first two and last two suffixes, respectively, these may be substituted each with one new suffix according to (7.3).
Further on the elements of the (cmn) matrix can be defined by the equation
| (7.9) |
 |
(7.10) |
| (7.11) |
Expressing the strains in terms of the stresses one obtains the equation
| (7.12) |
| (7.13) |
Simple calculation shows that between the elements of the above (smn)
matrix and the components of the [sijkl] tensor expressing the relationship
between the components of the [![]() ] strain and the
[
] strain and the
[![]() ] stress respectively the following correspondence holds
] stress respectively the following correspondence holds
 |
(7.14) |
Finally it should be noted that with the matrix notation, if applied, the number of the suffixes does not refer to the transformation formula of the matrix elements. Thus for example in case of a coordinate transformation the elements of the piezo-electric matrix (dij) transform differently from the matrix elements (cij) of the elasticity.
Copyright © 1984, 1998 International Union of Crystallography
IUCr Webmaster