4. Classification of Crystals

The abstractions of unit cells, lattice and symmetry to which reference has already been made lead to the possibility of classifying all crystals into well defined systems. On mathematical grounds it can be shown that there are only 7 possible unit-cell types (the 7 crystal systems) but, because some are capable of replicating themselves in a lattice in more than one way there are 14 possible lattices (the so-called Bravais lattices (Table 3)).

 

System Axial lengths and angles Bravais lattice Lattice symbol

Cubic Simple P

Body-centered I

Face-centered F

Tetragonal Simple P

Body-centered I

Orthorhombic Simple P

Body-centered I

Base-centered C

Face-centered F

Rhombohedral* Simple R

Hexagonal Simple P

Monoclinic Simple P

Base-centered C

Triclinic Simple P

* Also called trigonal.

There are 32 ways in which symmetry elements that can be detected by visual or morphological examination can be arranged (the 32 point groups) but, if all the symmetries possible on an atomic scale are included there are 230 possible arrangements (the 230 space groups).

It is interesting and important to remember that no crystal can have a 5-fold axis of symmetry. However, individual molecules can have such an axis of symmetry. A crystal cannot have a 5-fold axis because there is no way of packing by translation an infinite number of unit cells which have a pentagonal cross section so that they completely fill space.

Because space group symmetry is of little importance in the applications to be described, it will not be dealt with. Of course, a full understanding of the 230 space groups is necessary for anyone undertaking the determination of molecular structure by X-ray diffraction.

It will now be useful to consider the concept of families of planes running through a crystal and their labelling by the use of Miller indices. We will start by considering lines in two dimensions.

 

Figure 10

If we join the corners of the unit cells, or any other point in the cell, by straight lines we generate families of lines. All the lines in a family are parallel to each other and run through the same number of lattice points. Figure 10 shows three examples.

 

Figure 11

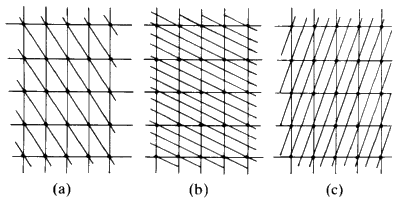Consider Fig. 11(a). Through each cell there is only one plane. Start from the lattice point at the bottom left hand corner, and consider that as the origin. You will travel along the vertical direction one cell edge before you encounter such a line; if you travel along the horizontal axis you will move one cell edge before you encounter a line. Consider the set of planes in sketch b. Start at the origin and move along the vertical axis. You will come in contact with three lines before you reach the first lattice point along that edge. If you move along the horizontal direction you will come in contact with only one line before you reach the first lattice point. In sketch c in moving along the vertical direction from the origin, to the first lattice point, you contact only one line; moving along the horizontal direction, you cross two lines. It should be fairly obvious that you can describe the geometry of these lines simply in terms of how many cut one unit cell edge or into what number of equal size pieces this family of lines will cut one unit cell edge. In sketch a, the family cuts the vertical axis into one and the horizontal axis into one. In sketch b, the vertical axis is cut into three, the horizontal axis, one. In sketch c, the vertical axis is cut once while the horizontal is cut twice. So the planes in sketch a could be labelled 1:1; those in b could be labelled 3:1, those in c could be labelled 1:2. The sign of the slope of the planes is obviously different between b and c and so we will have to define the positive and negative directions along the edges of the cell. Define the vertical direction, starting from the bottom left hand corner, going up as positive and horizontal, starting at the left hand corner, going across to the right as positive. We would then find that in a, you could number these planes as (+1 +1), in b they would be labelled (+3 +1) and in c the label would be (+1 -2). These numbers that can be assigned to the lines are called Miller Indices, and they are characteristic of these lines. It is obvious that the larger the Miller Indices that we can assign to the family of lines, the closer together these lines will be; in other words, the smaller will be the perpendicular distance between adjacent parallel lines. It should also be obvious that the distance between any pair of lines will be the same no matter where you are in the crystal because all the unit cells are the same size. What was done here in two dimensions is easily extended to three dimensions. Thus, any plane running through a crystal and passing through the set of lattice points--in other words through the corners of the unit cells--can be identified by a triplet of integers; the Miller Indices. The symbols used are the alphabetical letters (hkl). We can describe any plane in a crystal by three integers and these numbers can have + or - signs. A negative index, -n, is usually written as for convenience. Typical families of widely spaced planes might be: (110), 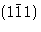etc.: planes such as (742), 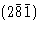etc., would be much closer together.

Copyright © 1981, 1997 International Union of Crystallography