2.1 Definition

This is an archive copy of the IUCr web site dating from 2008. For current content please visit https://www.iucr.org.

Next: 2.2 Fundamental law of the reciprocal Up: 2. Crystallographic Definition Previous: 2. Crystallographic Definition

2.1 Definition

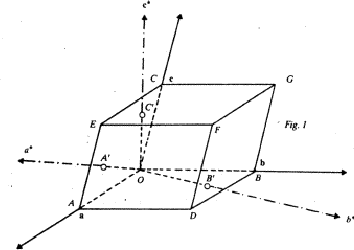
Let a, b, c be the basic vectors defining the unit cell of the direct lattice. The basic vectors of the reciprocal lattice are defined by:

$\begin{displaymath} \textbf{a*} = \frac{(\textbf{b} \wedge \textbf{c})}{(\textbf... ...c*} = \frac{(\textbf{a} \wedge \textbf{b})}{\textbf{(a, b, c)}}\end{displaymath}$ (2.1)

The modulus of a* is equal to the ratio of the area of the face OBCG opposite to a to the volume of the cell built on the three vectors a, b, c. Referring to Fig. 1, we may write:

$\begin{displaymath} \textbf{a*} = 1/OA^{\prime} \quad \textbf{b*} = 1/OB^{\prime} \quad \textbf{c*} = 1/OC^{\prime}\end{displaymath}$ (2.2)

$\begin{figure} \includegraphics {fig1.ps} \end{figure}$

From the definition of the reciprocal lattice vectors, we may therefore already draw the following conclusions:

(i) Each of the three vectors a*, b*, c* is normal to a set of lattice planes of the direct lattice (b, c; c, b; a, b) and their moduli are respectively equal to the inverse of the spacings of these three sets of lattice planes. The basic vectors of the reciprocal lattice possess therefore the properties that we were looking for in the introduction. We shall see in the next section that with each family of lattice planes of the direct lattice a reciprocal lattice vector may be thus associated.

(ii) The dimensions of the moduli of the reciprocal lattice vectors are those of the inverse of a length. For practical purposes the definition equations (2.1) may be rewritten after the introduction of a scale factor $\sigma$ which has the dimension of an area:

$\begin{displaymath} \textbf{a*} = \frac{(\textbf{b} \wedge \textbf{c})}{(\textbf{a, b, c})} \sigma\end{displaymath}$ (2.3)

This is only done to give the reciprocal lattice vector the dimension of length when one wants to actually draw the reciprocal lattice and we shall not make use of this scale factor in this paper.

From relations 2.1 it can readily be shown that the two sets of basic vectors satisfy the following equations:

$\begin{displaymath} \textbf{a}\cdot\textbf{a*} = 1\quad etc\dots \qquad \textbf{a}\cdot\textbf{b*} = 0 \quad etc\dots\end{displaymath}$ (2.4)

The two sets of equations (2.1) and (2.4) are equivalent and equations (2.4) are sometimes used as the definition equations of the reciprocal lattice. These relations are symmetrical and show that the reciprocal lattice of the reciprocal lattice is the direct lattice.

Next: 2.2 Fundamental law of the reciprocal Up: 2. Crystallographic Definition Previous: 2. Crystallographic Definition

IUCr Webmaster