3.3 Calculation of the reciprocal lattice vectors using the metric tensor

Relation (3.7) is the most convenient one to use to compute the reciprocal lattice parameters or any quantity related to them. Let a, b, c and $\alpha, \beta, \gamma$ be the direct lattice parameters. The doubly covariant coefficients of the metric tensor are then:

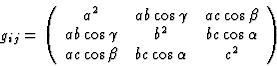$$g_{ij} = \left(\begin{array} {ccc} a^2 & ab\cos\gamma & ac \... ...os\alpha \\ ac\cos\beta & bc\cos\alpha & c^2\end{array} \right)$$ (3.15)

Its determinant, that is the square of the volume of the direct lattice unit cell is equal to:

$$V^2 = a^2b^2c^2(1+2 \cos \alpha \cos \beta \cos \gamma - \cos^2 \alpha - \cos^2 \beta - \cos^2 \gamma)$$ (3.16)

By inversing 3.15 we obtain the doubly contravariant of the metric tensor, g^ij

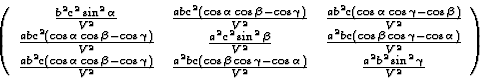$$\left(\begin{array} {ccc} \frac{b^2c^2 \sin^2 \alpha}{V^2} &... ...alpha)}{V^2}&\frac{a^2b^2\sin^2 \gamma}{V^2}\end{array} \right)$$ (3.17)

Using (3.17), we can easily obtain the following relations:

$$\textbf{a*} = \frac{b^2c^2 \sin^2 \alpha}{V^2} \textbf{a}+\f... ... - \cos \beta) \textbf{c}$$

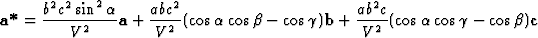
$$a* = \frac{bc \sin \alpha}{V}$$ (3.18)

$$\cos \gamma* = \frac{\cos \alpha \cos \beta - \cos \gamma}{\left\vert\sin \alpha \sin \beta \right\vert}$$

Copyright © 1981, 1998 International Union of Crystallography