4. Phase Restrictions

In the general case, where the contributions from the two parts of the unit cell have different amplitudes, F(hkl) can be represented as the sum of two vectors of different lengths (= amplitude) and different directions (= phase) as in Fig. 1.

**Figure 1:** The structure factor F(h) = F(*hkl*) represented as the vector sum of the contributions from two parts of the unit cell. In the general case these two parts differ both in amplitude and phase.
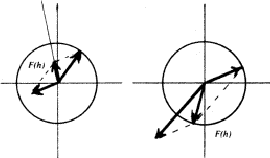$\begin{figure} \includegraphics {fig1.ps} \end{figure}$

The phase of F(hkl) can take on any value between 0 and 360 $^{\circ}$ . The special case that $\textbf{hR} = -\textbf{h}$ will result in a restriction of the possible value of the phase of F(hkl). This is called phase restriction. If the phase of the first contribution in (7) is $\alpha$ and ht = 0, then the phase of the second contribution becomes $-\alpha$ . As is clear from Fig. 2 the only possible phase values for the sum of these two contributions are 0 and 180 $^{\circ}$ , or if expressed in radians 0 and $\pi$ . If $-90 < \alpha < 90^{\circ}$ , the phase of F(hkl) becomes 0 $^{\circ}$ , and if $90 < \alpha < 270^{\circ}$ the phase of F(hkl) will be 180 $^{\circ}$ . The phase of Fhkl is denoted $\varphi$ here in order to distinguish it from the phase of the contributions.

**Figure 2:** In the special case where two halves of the unit cell have equal amplitude contribution, but opposite phases ( $\alpha$ and $-\alpha$ ), the resulting reflection will have a phase restriction.
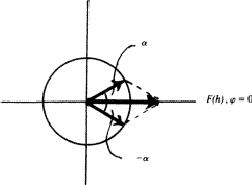$\begin{figure} \includegraphics {fig2.ps} \end{figure}$

All reflections in centrosymmetric space groups have phase restriction 0 ( $\pm$ 180 $^{\circ}$ ). Most reflections in non-centrosymmetric space groups lack phase restriction, but some special reflections have phase restriction. The phase is not necessarily restricted to 0 ( $\pm$ 180 $^{\circ}$ ) - such phase restrictions as 45 $^{\circ}$ , 60 $^{\circ}$ , 90 $^{\circ}$ and so on exist. All phase restrictions are $\pm$ 180 $^{\circ}$ or modulo 180 $^{\circ}$ . In order to clarify this an example is worked out in some detail.

What is the phase restriction of (h01) in space group P3₁21 (No 152)? The equivalent positions are:

$\begin{displaymath} (x, y, z),\quad (-y, x - y, \frac{1}{3} + z),\quad (y - x, -x, \frac{2}{3} + z),\end{displaymath}$

$\begin{displaymath} (y, x, -z),\quad (-x, y - x, \frac{1}{3} - z) \mbox{ and } (x - y, -y, \frac{2}{3} - z).\end{displaymath}$

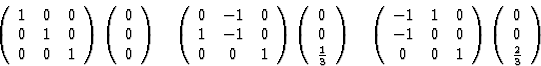
$\begin{displaymath} \left(\begin{array} {ccc} 1&0&0\\ 0&1&0\\ 0&0&1\end{array}\r... ...\left(\begin{array} {c}0\\ 0\\ \frac{2}{3}\end{array}\right)\\ \end{displaymath}$

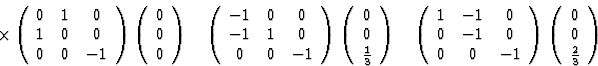
$\begin{displaymath} \times\left(\begin{array} {ccc}0&1&0\\ 1&0&0\\ 0&0&-1\end{ar... ...\left(\begin{array} {c}0\\ 0\\ \frac{2}{3}\end{array}\right)\\ \end{displaymath}$

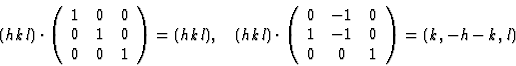
$\begin{displaymath} (hkl) \cdot \left(\begin{array} {ccc}1&0&0\\ 0&1&0\\ 0&0&1\e... ...ccc}0&-1&0\\ 1&-1&0\\ 0&0&1\end{array}\right) =(k, -h -k, l)\\ \end{displaymath}$

Phase restrictions occur if and only if (hkl) R_i = (-h, -k, -l), that is when the Friedel pair of a reflection is generated by any R_i. If $h \neq 0$ only R₅ creates a Friedel pair of (h01).

These results are introduced into (7). If the first summation over half the atoms in the unit cell gives a contribution to the structure factor of amplitude |F| and phase $\alpha$ , the other half of the atoms in the unit cell will give a contribution of amplitude |F| but with a phase exp (2 $\pi$ iht) $\cdot$ ( $-\alpha$ ). The value exp (2 $\pi$ iht) is short for exp (2 $\pi$ i[ht₁ + kt₂ + lt₃]) which in this case equals exp (2 $\pi$ i[ $h \cdot 0 + k \cdot 0 + 1 \cdot \frac{1}{3}$ ]) or exp (2 $\pi$ i/3) or +120 $^{\circ}$ (note the + sign!). The second sum thus has a phase of 120 $^{\circ}$ $-\alpha$ . As is clear from the geometrical interpretation in Fig. 3, the resulting structure factor will take on either the phase 60 $^{\circ}$ or 240 $^{\circ}$ .

**Figure 3:** A reflection with a phase restriction other than 0 or 180 $^{\circ}$ .
$\begin{figure} \includegraphics {fig3.ps} \end{figure}$

Reflections with phase restrictions are more often very strong or very weak than general reflections. This is due to the fact that the two contributions are either both large or both small in the case of a phase restricted reflection, whereas in the general case their amplitudes are independent. On the basis of their probability to take on extreme amplitude values all reflections are sorted into two categories: acentric or centric reflections. Reflections without phase restriction are called acentric and reflections with phase restrictions are called centric. The probability distribution of centric and acentric reflections is so different that it is often possible to distinguish between the space groups P1 and $P\overline{1}$ only from intensity data. The concept centric should not be mixed up with centrosymmetric or centred (also spelled centered). While centrosymmetric refers to a space group, centric refers only to single reflections. Although all reflections in centrosymmetric space groups are centric, not all reflections in non-centrosymmetric space groups are acentric.