Next: Suggestions for Further Reading Up: Rotation Matrices and Translation Vectors in Previous: 6. Systematic Enhancement, Epsilon
![[IUCr Home Page]](/iucr-top/logos/iucrhome.gif)
![[Commission Home Page]](/iucr-top/logos/cteach.gif)
![]()
![]()
![]()
Next: Suggestions for Further Reading
Up: Rotation Matrices and Translation Vectors in
Previous: 6. Systematic Enhancement, Epsilon
In analogy with equivalent positions, and as an effect of these, there are
equivalent reflections. Two equivalent reflections, h and
h![]() , always have the same amplitudes, i.e. |F(h)|
= |F(h
, always have the same amplitudes, i.e. |F(h)|
= |F(h![]() )|, but their phases may differ. The phases are,
however, related to each other in an easily deduced way. The difference in
phase between two equivalent reflections is called phase shift.
How the phase shift arises and how great it is will be shown now.
)|, but their phases may differ. The phases are,
however, related to each other in an easily deduced way. The difference in
phase between two equivalent reflections is called phase shift.
How the phase shift arises and how great it is will be shown now.
Two reflections h and h![]() are equivalent if there
exists an Ri such that
are equivalent if there
exists an Ri such that ![]() .Due to Friedel's law h is always equivalent to -h. The
phases of two equivalent reflections are related as:
.Due to Friedel's law h is always equivalent to -h. The
phases of two equivalent reflections are related as:
![]()
Proof :
The proof is carried out for a 2-fold symmetry for the sake of simplicity. A
similar strategy can be used for higher symmetries. If x and
Rx + t are equivalent positions, then R-1
(x - t) is also an equivalent position, since just as well
as x gives rise to x![]() , x
, x![]() gives
rise to x, by the same symmetry operation:
gives
rise to x, by the same symmetry operation:
![]()
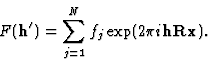 |
(8) |
![\begin{displaymath}
F(\textbf{h}^{\prime}) = \sum^{N/2}_{j=1} f_j \exp (2{\pi}i\...
...2{\pi}i\textbf{hR}[\textbf{R}^{-1}\{\textbf{x}-
\textbf{t}\}]).\end{displaymath}](img54.gif) |
(9) |
 |
(10) |
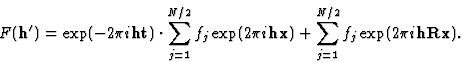 |
(11) |
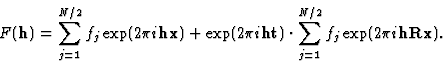 |
(7) |
The phase shift is called ![]() and is equal to
exp (
and is equal to
exp (![]() ht).
Note the minus sign!
ht).
Note the minus sign!
| (12) |
![]()
![]()
![]()
Note that the fifth reflection (![]() ) also is the Friedel pair of
(3 0 1).
Due to Friedel's law the phase of any reflection must be minus that of its
Friedel pair. In all cases where a symmetry operation generates an equivalent
reflection which is also its Friedel mate, we have two indications of the phase
value. If the phase of (hkl) is
) also is the Friedel pair of
(3 0 1).
Due to Friedel's law the phase of any reflection must be minus that of its
Friedel pair. In all cases where a symmetry operation generates an equivalent
reflection which is also its Friedel mate, we have two indications of the phase
value. If the phase of (hkl) is ![]() then the phase of (-h -k -l) is
then the phase of (-h -k -l) is
![]() due to Friedel's law, and the phase is
due to Friedel's law, and the phase is ![]() due to the
phase shift. We now have a system of equations:
due to the
phase shift. We now have a system of equations:
![]()
![]()
In a similar way the systematically absent reflections can be shown to be
exactly those reflections which have two contradictory phase indications. In
P21 (0 k 0)-reflections with k odd are extinct. The equivalent positions
of P21 are (x, y, z) and (![]() ). The reflections
(hkl) and (-h, k, -l) are equivalent and the phase shift is k/2. A
reflection like (0 3 0) is thus equivalent to itself, but the equivalent
reflection generated has a phase differing from the original one by
180
). The reflections
(hkl) and (-h, k, -l) are equivalent and the phase shift is k/2. A
reflection like (0 3 0) is thus equivalent to itself, but the equivalent
reflection generated has a phase differing from the original one by
180![]() . The phase of (0 3 0) is at the same time
. The phase of (0 3 0) is at the same time ![]() and
and ![]() , which of course is only possible if the amplitude of the
reflection is 0!
, which of course is only possible if the amplitude of the
reflection is 0!
Copyright © 1981, 1998 International Union of Crystallography
IUCr Webmaster