Letter to the Editor
Letter to the editor
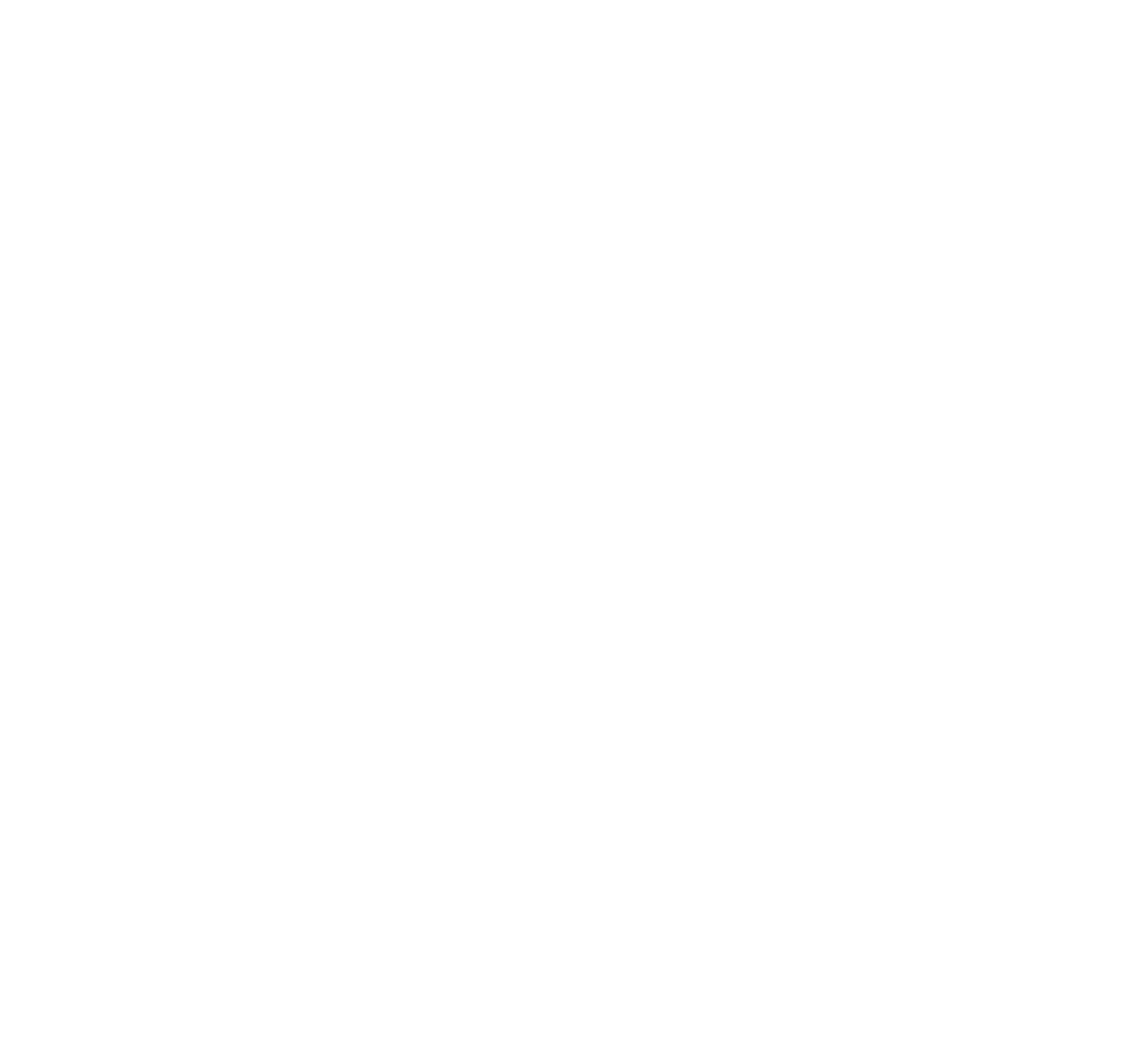
![[Cuprite graph]](https://www.iucr.org/__data/assets/image/0008/20420/cuprite.jpg) LAPW partial density of states at Cu in cuprite. Peaks indicate hybridization of 3dz2 partially unoccupied and holes in the experimental difference map. There's no evidence of hybridization of 4p, suggested by Wang and Schwartz (Angew Chem. Int Ed 2000, 39, 21)
LAPW partial density of states at Cu in cuprite. Peaks indicate hybridization of 3dz2 partially unoccupied and holes in the experimental difference map. There's no evidence of hybridization of 4p, suggested by Wang and Schwartz (Angew Chem. Int Ed 2000, 39, 21)
An exchange of letters between Spackman et al., Humphreys and Coppens (IUCr Newsletter, Vol. 8, Nos 1 and 3), commenting on our combined electron and X-ray diffraction measurement of charge-density in cuprite (Nature, 401, p. 49, 1999), raised three substantial points.
1. Accuracy of methods. Is kinematic X-ray diffraction sufficiently accurate to give meaningful charge densities? What standard is meaningful? If our goal is to understand bonding, we must compare experiment and theory. Due to recent progress, charge densities can be calculated with an accuracy comparable to the best experimental measurement [e.g. Pendellösung X-ray, Convergent-Beam Electron Diffraction (QCBED) data]. For the silicon standard, experiment and local density approximation (LDA) theory agree to within 0.24% (R factor), while LDA differs from Generalised Gradient Approximation theory by 0.1% (J. Phys. C9, 7541, 1997). The accuracy of kinematic X-ray work is limited by extinction. A detailed comparison of electron and X-ray data revealed that the error in the X-ray data, corrected for extinction, is about 1%, twice the structure factor difference between superimposed ions and the crystal for the three lowest order structure factors (Angew. Chem. Int. Ed. 2000, 39, p. 3791). We believe that this situation is typical for most inorganic materials referred to by Spackman, since the difference between results reported by different groups using different samples of the same material usually exceed experimental errors. The uncorrectable errors are due to statistical variation in the mosaic block distribution, and differences in crystal samples. QCBED patterns are taken from volumes of the order of 104 nm3, much smaller than one 'mosaic block', allowing use of the many-beam perfect crystal theory. Electron diffraction also benefits from the fact that electron intensities increase inversely as the scattering angle to the fourth power for the low angle bond-sensitive reflections. Our results for low order in MgO (P.R. L. 78, p. 4777, 1998), NiO (Phys. Rev. B61, p. 2506, 2000) and cuprite are the most accurate, lie closest to ab-initio band-structure calculations, and, with the Pendellösung results, are indeed the only ones capable of distinguishing many-electron approximations in the theory. The MgO work achieved a tenfold improvement in accuracy over previous X-ray work. The critical voltage method (Smart and Humphreys Inst. Phys. Conf. Ser. 52, p. 211, 1980) provides comparably accurate ratios of structure factors. Three-phase invariants may be measured by many-beam electron diffraction with better than one degree accuracy in phase (Acta Cryst. A49, 422, 1993), P.R.L. 62, 547, 1989).
2. Use of the term 'orbital'. We agree with Scerri (J. Chem. Ed. 77, p. 1492, 2000) that orbital wavefunctions are unobservable and that 'the orbital model remains enormously useful...and lies at the heart of much of computational chemistry; but it is just that, a model'. Aware of this, we use many-electron theory in all the calculations reported in our paper. However, it would be perverse not to mention the resemblance of the shape of the hole in our difference map to the simple one-electron model of a d-orbital charge-density distribution. This similarity indicates that the one-electron model retains validity, even in a transition metal oxide, and that one particular choice of basis functions converges more efficiently than others. Continued use of terms and concepts such as 'd-electron', 'molecular orbital diagram', 'orbital degeneracy', 'orbital angular momentum', 'anti-bonding orbital' etc. by workers in the field suggests that one-electron theory is too useful an approximation to be abandoned.
3. The significance of difference charge density. Our charge density image shows the difference between the experiments and the spherical ion model of Cu+ and O2-. the negative difference charge density around Cu is due to the dz2 hole formed by 3dz2 and 4s hybridization, a picture supported by both theory and experiment (Marksteiner et al., Zeit. Phys. B64, 119 (1989); Grioni et al., Phys. Rev. B45, 3309 (1992)). The figure above shows the partial density of states around Cu calculated by the LAPW method. Restori and Schwarzenbach, (Acta Cryst. B42, 201, (1986)) show that in the molecular-orbital model the charge density around Cu is a sum of partially occupied |ψz2|2 and |ψ|2, other fully-occupied d-states and the interference terms, Re(ψz2ψs) and Re(ψxzψyz). These, when subtracted from the charge density of a closed-shell Cu+ with a fully occupied dz2 state, would give a negative charge density for |ψz2|2. The resemblance of our difference map to the dz2 orbital arises from the fact that the radial distributions are very different for the dz2 (<1) and 4s (>1) electrons, thus spatially separating the dz2 and 4s electrons. The agreement between this single-electron model and our experiment, supported by many-electron calculations and spectroscopic evidence, vindicates the quantum mechanical single electron approximation for this case.
In closing, we note that while the electron community has consistently taken full account of X-ray works (which is vital, since QCBED remains inaccurate for high orders and temperature factors), this practice has rarely been reciprocated. It seems clear that both methods are needed for greatest accuracy, especially for high symmetry inorganic materials with small unit cells.
J.C.H. Spence, J.M. Zuo* and M. O'Keefe ASU (spence@asu.edu)*UIUC (jianzuo@uiuc.edu)