2. The Properties of the Stereographic Projection

This is an archive copy of the IUCr web site dating from 2008. For current content please visit https://www.iucr.org.

There is, of course, a variety of different projections of the sphere on to a plane that could be used to represent the angular relations between the poles of the faces of a crystal. However, what makes the stereographic projection pre- eminently suitable for this purpose is the fact that it projects any circle on the sphere into a circle on the projection (although in some cases this circle becomes of infinite radius, i.e. a straight line).

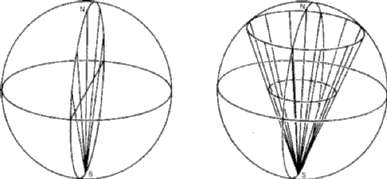
It is evident from Fig. 1 that if P were to lie on the equator of the sphere then p would coincide with it. In other words the equator coincides with its own projection. The circle on the stereographic projection which represents the equator of the sphere plays an important role and is called the primitive of the projection. Angular distances round the equator on the sphere obviously project to identical angular distances round the primitive. It is also clear from Fig. 1 that the north pole of the sphere projects to the centre of the primitive. Figure 3 shows that a meridian of the sphere projects to a diameter of the primitive (this is the case where the projection is a circle of infinite radius), and a circle of latitude on the northern hemisphere projects to a circle concentric with the primitive and inside it. If the circle of latitude were in the southern hemisphere it would project to a concentric circle outside the primitive.

**Figure 3:** (a) A meridian on the sphere projects into a diameter of the primitive. (b) A circle of latitude projects into a circle concentric with the primitive.
$\begin{figure} \includegraphics {fig3.ps} \end{figure}$

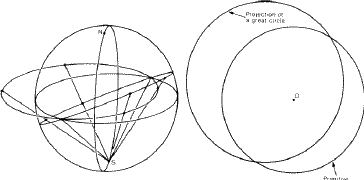
The equator and a meridian are special kinds of great circles on the sphere. A great circle in a general position is shown in Fig. 4, and it is clear that its stereographic projection is a circle of radius greater than that of the primitive. It intersects the latter in two points at opposite ends of a diameter of the primitive. The half of the great circle which is in the northern hemisphere projects to the arc that is within the primitive, and the half in the southern hemisphere projects to the rest of the circle outside the primitive. For some purposes (as discussed in Section 3) it is necessary to consider the whole circle, but for other purposes it is more convenient to project the half-circle in the southern hemisphere to the north pole so as to confine the projection within the primitive. When this is done the complete representation of the great circle consists of two arcs of large radius symmetrically related across a diameter of the primitive as shown in Fig. 5.

**Figure 4:** A great circle in a general position in the sphere projects into a circle that intersects the primitive at opposite ends of a diameter; (a) perspective view; (b) the projection itself.
$\begin{figure} \includegraphics {fig4.ps} \end{figure}$

The equator and circles of latitude are of course centred at the north pole, and their projections are also centred at the projection of the north pole, that is at the centre of the primitive. However this is the only case in which the projection of the centre of a circle coincides with the centre of the projection of the circle. This is evident for great circles from Fig. 4; that it is also true for small circles will be evident from Section 3(iv) below.

**Figure 5:** The broken line shows the portion of the projection of the great circle of Fig. 4 that lies outside the primitive, reprojected to the N-pole.
$\begin{figure} \includegraphics {fig5.ps} \end{figure}$