3. Geometrical Construction of a Stereographic Projection

There are four important constructions that can be done very easily on the stereographic projection with the aid of a ruler, compasses and protractor.

(i) To plot the projection of a point at a given angle $\theta$ from the N- pole. Consider a meridional section of the sphere through the point P as in Fig. 6a. The point P can be inserted (using the protractor) and joined to S. It cuts the equatorial section at p. A circle of radius Op can then be transferred to the projection (Fig. 6b) and this circle describes the projections of all points at $\theta$ from the N-pole. If the orientation of the meridian on which P lies has already been fixed on the stereogram then p is the point where this intersects the circle; if not already fixed by other considerations such a meridian can be drawn in as a diameter of the primitive in an arbitrary orientation.


 
Figure 6: Construction of the stereographic projection of a point that lies at an angle $\theta$ from the N-pole: (a) construction on vertical section of the sphere; (b) the projection.
\begin{figure} \includegraphics {fig6.ps} \end{figure}

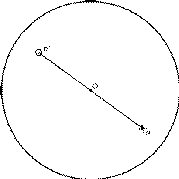
(ii) To plot the projection of the opposite of a given point.

On the meridional section of the sphere through the point P (Fig. 7a) it is easy to join P to the centre and extend it to the opposite point $P^{\prime}$ (its antipodes). The projection of $P^{\prime}$ to the S-pole then gives its projection $p^{\prime}$ at a distance $Op^{\prime}$ from the centre. If p has already been plotted on the stereograph (Fig. 7b) then it can be joined to the centre O and this line continued a distance $Op^{\prime}$ transferred from Fig. 7a.


 
Figure 7: Construction of the stereographic projection $p^{\prime}$ of the opposite of a pole whose projection is p: (a) construction on vertical section of the sphere; (b) the projection.
\begin{figure} \includegraphics {fig7.ps} \end{figure}

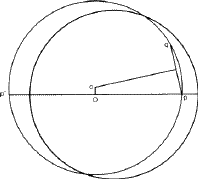
For the following construction (iii) we shall require the opposite of P projected to the S-pole in this way. However, if, for other purposes, we require to project the opposite of P to the N-pole then the procedure is very simple; on this convention $p^{\prime}$ is simply obtained by joining p to the centre of the stereogram and continuing this line for an equal distance beyond the centre to make $Op^{\prime}=Op$ as in Fig. 8.


 
Figure 8: Projection ($p^{\prime}$) to the N-pole of the opposite of a point whose projection (to the S-pole) is p.
\begin{figure} \includegraphics {fig8.ps} \end{figure}

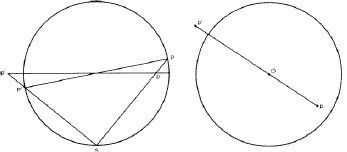
(iii) to draw the projection of a great circle through any two points. Let the projections of the two points P and Q be p and q (Fig. 9). Since every great circle through any point passes through the opposite of that point, the great circle through P and Q must pass through $P^{\prime}$, the opposite of P. Thus the required projection is the circle through $pp^{\prime}q$, and $p^{\prime}$ is found by means of construction (ii). The centre of this circle is at the intersection of the perpendicular bisectors of pq and $pp^{\prime}$, and the circle can therefore be drawn.


 
Figure 9: Construction of the projection of a great circle through two points whose projections are p and q.
\begin{figure} \includegraphics {fig9.ps} \end{figure}

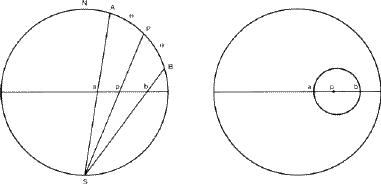
(iv) To draw the projection of a small circle of radius $\theta$ from a given point. Such a circle is the locus of all points at an angular distance $\theta$ from the given point P, and is therefore very useful when we wish to plot a point at some specified angle from another point. Consider the meridional section of the sphere through P (Fig. 10a). Mark off two points, A and B at angular distances $\theta$ at each side of P. Join A, B and P to S to find their projections a, b and p. Transfer to the stereogram distances ap and bp along the diameter through p. Then a and b obviously lie on the required circle and its centre must be the mid-point of ab. (Note that this centre is displaced outwards from p itself.) The circle can therefore be drawn directly (Fig. 10b).


 
Figure 10: Construction of the projection of a small circle of radius $\theta$ round a point P: (a) construction on vertical section of the sphere; (b) the projection.
\begin{figure} \includegraphics {fig10.ps} \end{figure}

It is commonly required to find a point at a given angle from p and at some other given angle from q. Such a point is at the intersection of the two corresponding small circles. There are of course two such points of intersection, but there will usually be some qualitative reason to show which of these two points is the one required.

All the four procedures described above have involved a subsidiary drawing of a meridional section of the sphere; the actual construction has been performed on this section, and appropriate measurements have been transferred from it to the stereogram itself. This use of a subsidiary drawing is helpful in clarifying the principles involved, but it is not necessary in practice. If the meridional section is imagined to be rotated by 90$^{\circ}$ about its equatorial diameter then it coincides with the plane of the stereogram, so that the construction lines can be drawn on the stereogram itself. If they are erased afterwards then the same result is obtained directly without having to transfer a measurement. This way of carrying out construction (ii) is shown by broken lines in Fig. 11, and the method is equally applicable to all the constructions.


 
Figure 11: Construction as in Fig. 7a carried out on the stereographic projection itself
\begin{figure} \includegraphics {fig11.ps} \end{figure}

next up previous
Next: 4. The Use of Stereographic Nets Up: The Stereographic Projection Previous: 2. The Properties of the Stereographic

Copyright © 1984, 1997 International Union of Crystallography

IUCr Webmaster