1. Simple Symmetry Operations

The general idea of symmetry is familiar to almost everyone. Formally it can be defined in various ways. The Concise Oxford Dictionary says `1. (Beauty resulting from) right proportion between the parts of the body or any whole, balance, harmony, keeping. 2. Such structure allows of an object's being divided by a point or line or plane or radiating lines or planes into two or more parts exactly similar in size and shape and in position relative to the dividing point, etc., repetition of exactly similar parts facing each other or a centre,...'.

The second of the definitions is the one that relates most closely to crystallography and thus concerns us here, but I have also included the first because it seems to me to express just why the subject is both satisfying and enjoyable. Symmetry and art go hand in hand, as in Fig. 1.1. However, the symmetry of Fig. 1.1 is much more complicated than appears on first sight, so we will begin by considering something rather simpler.

**Fig. 1.1.** Pattern based on a fourteenth-century Persian tiling design.
$\begin{figure} \includegraphics {fig1.1.ps} \end{figure}$

A teacup will do for a start (Fig. 1.2). This is an example of an object that can be divided into two parts by a plane. Since the two parts are mirror images of one another, this symmetry element is called a mirror plane . Operation of this element on one half of the teacup generates the other: if a half teacup is held with its sliced edge against a mirror, the appearance of the whole is regenerated. Teacups are rarely sliced in real life (although it was done in the cartoon version of `Alice in Wonderland'), but you could try it out with an apple or a pear. If the two similar parts produced have no symmetry remaining, as in the case of the teacup, they are called asymmetric units .

**Fig. 1.2.** A teacup, showing its mirror plane of symmetry. (After L. S. Dent Glasser, Crystallography & its applications: Van Nostrand Reinhold, 1977.)
$\begin{figure} \includegraphics {fig1.2.ps} \end{figure}$

Biological objects such as flowers frequently show symmetry. The person -- hereafter referred to as `it' -- shown in Fig. 1.3a also has a mirror plane, provided it stands and parts its hair in the middle (and that we ignore its internal organs). Each half of the figure is an asymmetric unit. Moving an arm or leg destroys the symmetry and the whole figure can then be treated as an asymmetric unit. We will use this little person, both with and without its mirror plane, to illustrate further symmetry elements, and to build more complicated groups.

If the figure holds hands with its identical twin, as in Fig. 1.3b, the group formed no longer has a mirror plane. On the other hand rotating the group through 180 $^{\circ}$ about an axis (indicated in the diagram) brings each figure into coincidence with its twin. This group has an axis of rotation , twofold in this case because the operation has to be performed twice before each figure returns to its original position. Fig. 1.3c shows how a different method of holding hands produces a group that combines mirror planes with a twofold axis; in Fig. 1.3d identical triplets demonstrate a threefold axis. (Can you identify a fourfold axis in Fig. 1.1? Does it have any two- fold axes or mirror symmetry?)

**Fig. 1.3.** Some symmetry elements, represented by human figures. (a) Mirror plane, shown as dashed line, in elevation and plan. (b) Twofold axis, lying along broken line in elevation, passing perpendicularly through clasped hands in plan. (c) Combination of twofold axis with mirror planes; the position of the symmetry elements given only in plan. (d) Threefold axis, shown in plan only. (e) Centre of symmetry (in centre of clasped hands). (f) Fourfold inversion axis, in elevation and plan, running along the dashed line and through the centre of the clasped hands. (After L. S. Dent Glasser, Chapter 19, The Chemistry of Cements: Academic Press, 1964.)
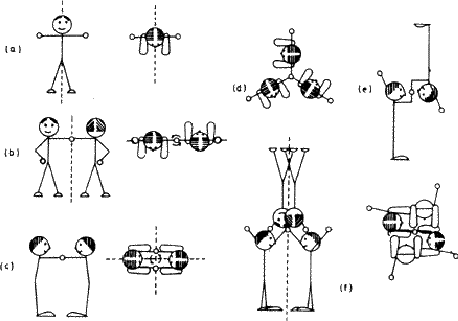$\begin{figure} \includegraphics {fig1.3.ps} \end{figure}$

Isolated objects or groups of objects may show any number of mirror planes and any kind of axis; the symmetry of an infinite array of identical groups, such as is found in a crystal, is limited by having to pack the units together in three dimensions. This limitation means that in crystallography only centres of symmetry (Fig. 1.3e), mirror planes, two-, three-, four- and sixfold and the corresponding inversion axes are encountered. An inversion axis involves rotation plus inversion through a point; Fig. 1.3f represents a fourfold inversion axis, rotation through one fourth of a revolution being followed by inversion through a point in the middle of clasped hands. A onefold inversion axis is equivalent to a centre of symmetry (Fig. 1.3e) and a twofold inversion axis to a mirror plane. (This last equivalence is important in other contexts because it established a mirror plane as a twofold symmetry operator.)

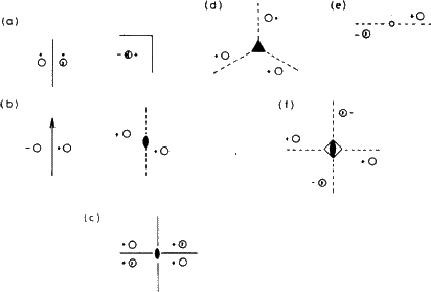
It is not very convenient to illustrate symmetry elements in the way that we have just used: rather than drawing little people we use circles to represent asymmetric units: conventionally an open circle represents a right-handed unit and a circle with a comma in its mirror image or enantiomorph (i.e. a left-handed unit). Fig. 1.4 shows the same groups as Fig. 1.3, represented in this formal, shorthand way.

**Figure 1.4.** The arrangements in Fig. 1.3 redrawn using conventional symbols. The right-hand group of (a) is drawn here in a different orientation, and the left-hand groups of (c) and (f) are omitted. Symbols + and - represent equal distances above and below the plane of the paper: open circles represent asymmetric units of one hand, and circles with commas their enantiomorphs. (a) Mirror plane (m), perpendicular to (left) and in the plane of the paper. (b) Twofold axis (2) in the plane of the paper (left) and perpendicular to it (right). (c) Combination of twofold axes and mirror planes. Note that the presence of any two of these elements creates the third. (d) Three fold axis (3). (e) Centre of symmetry (1). (f) Fourfold inversion axis ( $\bar 4$ ).
$\begin{figure} \includegraphics {fig1.4.ps} \end{figure}$

Even this is inconvenient in written text, in which mirror planes are given the symbol m, while axes and the corresponding inversion axes are referred to as $1, \bar{1}; 2, \bar{2} (\equiv m); 3, \bar{3}; 4, \bar{4}; 6, \bar{6}$ . The symbol 1 (for a onefold axis) means no symmetry at all, while the corresponding inversion axis ( $\bar{1}$ ) is equivalent, as already remarked, to a centre of symmetry.