Next: 6. Higher Order Effects Up: An Introduction to Crystal Physics (Description Previous: 4. Neumann's Principle
![[IUCr Home Page]](/iucr-top/logos/iucrhome.gif)
![[Commission Home Page]](/iucr-top/logos/cteach.gif)
![]()
![]()
![]()
Next: 6. Higher Order Effects
Up: An Introduction to Crystal Physics (Description
Previous: 4. Neumann's Principle
One frequently finds data in the literature which give the value of some physical property in a given direction. In this chapter the concept of the magnitude of a physical property in a given direction, and also the relationship between this value and the respective tensor components will be illustrated on the examples of the direction dependence of the electrical conductivity and Young's modulus respectively.
The specific conductivity in the direction of the electric field is defined as
the ratio of the component parallel with the electric field of the current
density (![]() ) and the magnitude of the electric field (E), i.e.
) and the magnitude of the electric field (E), i.e.
![]() . Let the components of the electric field be Ei = Eni,
where ni denotes the i-th component of the unit vector (
. Let the components of the electric field be Ei = Eni,
where ni denotes the i-th component of the unit vector (![]() ) pointing
into the direction of the electric field. The component of the j current
density parallel with
) pointing
into the direction of the electric field. The component of the j current
density parallel with ![]() is in tensor notation
is in tensor notation
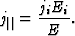 |
(5.1) |
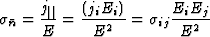 |
(5.2) |
| (5.3) |
Equation (5.3) may be applied in two ways. One possibility is to calculate the tensor components from the measured conductivity values and the corresponding direction cosines. For this purpose one should measure the electrical conductivity in different directions, which are not connected by symmetry, as many times as the number of the independent components. Another possibility of applying eq. (5.3) is quite opposite to the first one. With the aid of the already known tensor components the conductivity value can be computed for any direction.
Equation (5.3) becomes considerably simplified for crystals of the tetragonal,
trigonal and hexagonal systems which have only two independent tensor-components
(![]() and
and ![]() )
)
| (5.4) |
| (5.5) |
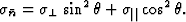 |
(5.6) |
As another example we will study the direction dependence of Young's modulus.
To begin with it should be stated that Young's modulus in the pulling direction
is defined as the ratio of the longitudinal stress (![]() ) and the
longitudinal strain (
) and the
longitudinal strain (![]() . If the
. If the ![]() axis of the
coordinate system is placed in the direction of the
axis of the
coordinate system is placed in the direction of the ![]() unit vector Young's
modulus in this direction will apparently be
unit vector Young's
modulus in this direction will apparently be
| (5.7) |
| (5.8) |
| (5.9) |
| (5.10) |
| (5.11) |
| (5.12) |
Copyright © 1984, 1998 International Union of Crystallography
IUCr Webmaster