This is an archive copy of the IUCr web site dating from 2008. For current content please visit
https://www.iucr.org.
![[IUCr Home Page]](/iucr-top/logos/iucrhome.gif)
![[Commission Home Page]](/iucr-top/logos/cteach.gif)




Next: Crystallographic groups
Up: Crystallographic symmetry
Previous: Crystallographic site-symmetry operations
Space-group operations
The following facts are stated, their proof is beyond the scope of this
manuscript:
- The symmetry operations listed in Section 3.2 are elements of
space groups which leave the given point
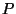 fixed, see definition
(D 3.2.1). Therefore, identity, inversion, rotations, rotoinversions,
and reflections are symmetry operations of space groups.
Moreover, the same restrictions for the possible angles of rotation and
rotoinversion of space-group operations hold as in Section 3.2.
This concerns also the rotations involved in screw rotations.
fixed, see definition
(D 3.2.1). Therefore, identity, inversion, rotations, rotoinversions,
and reflections are symmetry operations of space groups.
Moreover, the same restrictions for the possible angles of rotation and
rotoinversion of space-group operations hold as in Section 3.2.
This concerns also the rotations involved in screw rotations.
- It is always possible to choose a primitive basis,
see definition (D 1.5.2) and the remarks to it. Referred to a primitive
basis, all lattice vectors of the crystal are integer linear combinations
of the basis vectors. Each of these lattice vectors defines a (symmetry)
translation. The order of any translation T is infinite
because there is no number
 such that
such that
 .
.
- Parallel to each rotation, screw-rotation, or rotoinversion axis
as well as parallel to the normal of each mirror or glide plane there
is a row of lattice vectors.
- Perpendicular to each rotation, screw-rotation, or rotoinversion
axis as well as parallel to each mirror or glide plane there is a
plane of lattice vectors.
- Let
 be the rotation angle of a screw rotation,
then the screw rotation is called
be the rotation angle of a screw rotation,
then the screw rotation is called 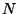 -fold. Note that the order of any
screw rotation is infinite. Let u be the shortest lattice vector
in the direction of the corresponding screw axis, and
-fold. Note that the order of any
screw rotation is infinite. Let u be the shortest lattice vector
in the direction of the corresponding screw axis, and
 ,
with
,
with  and integer, be the screw vector of the
screw rotation by the angle
and integer, be the screw vector of the
screw rotation by the angle  . Then the
. Then the  symbol of the screw
rotation is
symbol of the screw
rotation is  .
.
Performing an 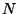 -fold rotation
-fold rotation 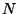 -times results in the
identity mapping, i.e. the crystal has returned to its
original position. After
-times results in the
identity mapping, i.e. the crystal has returned to its
original position. After 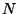 screw rotations with
rotation angle
screw rotations with
rotation angle
 the crystal has its original
orientation but is shifted parallel to the screw axis by the lattice
vector
the crystal has its original
orientation but is shifted parallel to the screw axis by the lattice
vector 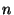 u.
u.
- Let W be a glide reflection.
Then the glide vector is
parallel to the glide plane and is 1/2 of a lattice vector t.
Whereas twice the application of a reflection restores the
original position of the crystal, applying a glide reflection
twice results in a translation of the crystal with the translation
vector t. The order of any glide reflection is infinite. The
 symbol of a glide reflection is
symbol of a glide reflection is 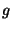 in the plane and
in the plane and
 , or
, or 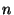 in the space. The letter indicates the direction of the
glide vector g relative to the basis of the coordinate system.
in the space. The letter indicates the direction of the
glide vector g relative to the basis of the coordinate system.




Next: Crystallographic groups
Up: Crystallographic symmetry
Previous: Crystallographic site-symmetry operations
Copyright © 2002 International Union of
Crystallography
IUCr
Webmaster
![]() -fold rotation
-fold rotation ![]() -times results in the
identity mapping, i.e. the crystal has returned to its
original position. After
-times results in the
identity mapping, i.e. the crystal has returned to its
original position. After ![]() screw rotations with
rotation angle
screw rotations with
rotation angle
![]() the crystal has its original
orientation but is shifted parallel to the screw axis by the lattice
vector
the crystal has its original
orientation but is shifted parallel to the screw axis by the lattice
vector ![]() u.
u.
![[IUCr Home Page]](/iucr-top/logos/iucrhome.gif)
![[Commission Home Page]](/iucr-top/logos/cteach.gif)