Crystallographic groups

The symmetry, i.e. the set of all symmetry operations, of any object forms a group in the mathematical sense of the word. Therefore, the theorems and results of group theory can be used when dealing with the symmetries of crystals. The methods of group theory can not be treated here but a few results of group theory for crystallographic groups will be stated and used.

We start with the definition of the terms `subgroup' and `order of a group'.

Definition (D 3.4.3) Let $\mbox{$\mathcal{G}$}$ and $\mbox{$\mathcal{H}$}$ be groups such that all elements of $\mbox{$\mathcal{H}$}$ are also elements of $\mbox{$\mathcal{G}$}$. Then $\mbox{$\mathcal{H}$}$ is called a subgroup of $\mbox{$\mathcal{G}$}$.

Remark. According to its definition, each crystallographic site-symmetry group is a subgroup of that space group from which its elements are selected.

Definition (D 3.4.3) The number $g$ of elements of a group $\mbox{$\mathcal{G}$}$ is called the order of $\mbox{$\mathcal{G}$}$. In case $g$ exists, $\mbox{$\mathcal{G}$}$ is called a finite group. If there is no (finite) number $g$, $\mbox{$\mathcal{G}$}$ is called an infinite group.

Remark. The term `order' is an old mathematical term and has nothing to do with order or disorder in crystals. Space groups are always infinite groups; crystallographic site-symmetry groups are always finite.

The following results for crystallographic site-symmetry groups $\mbox{$\mathcal{S}$}$ and point groups $\mbox{$\mathcal{P}$}$ are known for more than 170, those for space groups $\mbox{$\mathcal{R}$}$ more than 100 years.

We consider site-symmetry groups first.

1. The possible crystallographic site-symmetry groups $\mbox{$\mathcal{S}$}$ are always finite groups. The maximal number of elements of $\mbox{$\mathcal{S}$}$ in the plane is 12, in the space is 48.
2. Due to the periodicity of the crystal, crystallographic site-symmetry groups never occur singly. Let $\mbox{$\mathcal{S}$}$ be the site-symmetry group of a point $P$, and $P'$ be a point which is equivalent to $P$ under a translation of $\mbox{$\mathcal{R}$}$. To $P'$ belongs a site-symmetry group $\mbox{$\mathcal{S}$}'$ which is equivalent to $\mbox{$\mathcal{S}$}$. The infinite number of translations results in an infinite number of points $P'$ and thus in an infinite number of groups $\mbox{$\mathcal{S}$}'$ which all are equivalent to $\mbox{$\mathcal{S}$}$. In Subsection 5.3.1 is shown, how $\mbox{$\mathcal{S}$}'$ can be calculated from $\mbox{$\mathcal{S}$}$.

   Note that this assertion is correct even if not all of the groups $\mbox{$\mathcal{S}$}'$ are different. This is demonstrated by the following example: If the site symmetry $\mbox{$\mathcal{S}$}$ of $P$ consists of a reflection and the identity, the point $P$ is placed on a mirror plane. If the translation mapping $P$ onto $P'$ is parallel to this plane, then $\mbox{$\mathcal{S}$}$ of $P$ and $\mbox{$\mathcal{S}$}'$ of $P'$ are identical. Nevertheless, there are always translations of $\mbox{$\mathcal{R}$}$ which are not parallel to the mirror plane and which carry $P$ and $\mbox{$\mathcal{S}$}$ to points $P''$ with site symmetries $\mbox{$\mathcal{S}$}''$. These are different from but equivalent to $\mbox{$\mathcal{S}$}$. The groups $\mbox{$\mathcal{S}$}$ and $\mbox{$\mathcal{S}$}''$ leave different planes invariant.

3. According to their geometric meaning the groups $\mbox{$\mathcal{S}$}$ may be classified into types. A type of site-symmetry groups is also called a crystal class.
4. There are altogether 10 crystal classes of the plane. Geometrically, their groups are the symmetries of the regular hexagon, of the square, and the subgroups of these symmetries. Within the same crystal class, the site-symmetry groups consist of the same number of rotations and reflections and have thus the same group order. The rotations have the same rotation angles. Site-symmetry groups of different crystal classes differ by the number and angles of their rotations and/or by the number of their reflections and often by their group orders.
5. There are 32 crystal classes of groups $\mbox{$\mathcal{S}$}$ of the space. Their groups are the symmetries of the cube, of the hexagonal bipyramid, and the subgroups of these symmetries. Again, the groups $\mbox{$\mathcal{S}$}$ of the same crystal class agree in the numbers and kinds of their rotations, rotoinversions, reflections, and thus in the group orders. Moreover, there are strong restrictions for the possible relative orientations of the rotation and rotoinversion axes and of the mirror planes. Site-symmetry groups of different crystal classes differ by the numbers and kinds of their symmetry operations.
6. In order to get a better overview, the crystal classes are further classified into crystal systems and crystal families.

The following exercise deals with a simple example of a possible planar crystallographic site-symmetry group.

Problem 1A. Symmetry of the square.

For the solution, see p. .

Fig. 3.4.1 The vertices 1, 2, 3, 4 of the square are described by their coordinates 1,1; -1,1; -1,-1; 1,-1, respectively. The coordinates are referred to the axes a and b and to the center of the square as origin.

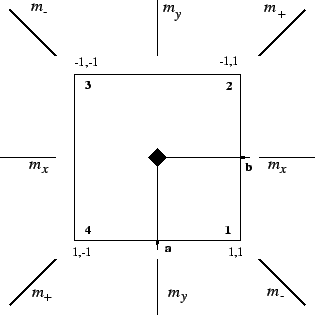Questions For further questions, see Problem 1B, p. .

(i)

List the symmetry operations of the square.

(ii)

What is the geometric meaning of each of these symmetry
operations ?

(iii)

What are the orders of these symmetry operations ?

(iv)

How many symmetry operations of the square do exist ?

Some remarks on space groups follow.

Space groups are the symmetries of crystal patterns, they have been defined already by definition (D 3.2.1). Their order is always infinite because of the infinitely many translations. Not only the order but also the number of space groups is infinite because each existing or conceivable crystal (crystal pattern) has `its' space group. However, an infinite set, as that of all space groups, is difficult to overlook. Therefore, it is advantageous to have a classification of the space groups into a finite number of classes.

The classification of site symmetries into types of site symmetries (crystal classes) has already been discussed. Like site-symmetry groups, also space groups may be classified into types, the space-group types. This classification into 230 space-group types is so commonly used that these space-group types are just called the 230 space groups in many text books and in the spoken language. In most cases there is no harm caused by this usage. However, for certain kinds of problems in crystal chemistry, or when dealing with phase transitions, the distinction between the individual `space group' and the set `type of space groups' is indispensable. The distinction is important enough to be illustrated by an example from daily life:

There are millions of cars running on earth but there are only a few hundred types of cars. One loosely says: `I have the same car as my neighbour' when one means `My car is of the same type as that of my neighbour'. The difference becomes obvious if the neighbour's car is involved in a traffic accident.

Really, there are 2 classifications of space groups into types. The one just mentioned may be called the `classification into the 230 crystallographic space-group types'. The different types are distinguished by the occurence of different types of rotations, screw rotations, etc. (One can not argue with the `numbers of 2-fold rotations' etc. because in space groups all these numbers are infinite). However, there are 11 pairs of these types, called enantiomorphic pairs, where in each pair the space groups of the one type can be transferred to those of the other type by improper but not by proper mappings. (Proper and improper mappings are defined in analogy to the proper and improper isometries, see Section 3.1. A pair of enantiomorphic space-group types is analogous to a pair of gloves: right and left). Counting each of these pairs as one type results in altogether 219 affine space-group types.

More than 2/3 of the 878 pp. of Vol. IT A, 4th edition (1995) are devoted to the description of the 17 `plane groups' and the 230 `space groups' (really: plane-group and space-group types). There are 4 ways for this description; 2 of them are described in the next section, the others in Sections 4.6 and 5.2.

The term point-symmetry group, point group, or point symmetry $\mbox{$\mathcal{P}$}$ is used in 2 different meanings. In order to have a clear distinction between the 2 items which are commonly called `point symmetry', the one item has been called `site-symmetry group' $\mbox{$\mathcal{S}$}$ or `site symmetry', see above. This is done also in IT A, Section 8, `Introduction to space-group theory'. The other item is the external symmetry $\mbox{$\mathcal{P}$}$ of the ideal macroscopic crystal. It is simultaneously the symmetry of its physical properties. The symmetry $\mbox{$\mathcal{P}$}$ is very much related to the symmetry $\mbox{$\mathcal{S}$}$ in so far as to each group $\mbox{$\mathcal{S}$}$ there exists a group $\mbox{$\mathcal{P}$}$ with the same order, the same number and kind of rotations, rotoreflections, and reflections, although not necessarily in the same space group. Analogously, to each group $\mbox{$\mathcal{P}$}$ there may exist groups $\mbox{$\mathcal{S}$}$ which have the same `structure' as $\mbox{$\mathcal{P}$}$ has. Taken as groups without paying attention to the kind of operations, $\mbox{$\mathcal{S}$}$ and $\mbox{$\mathcal{P}$}$ cannot be distinguished. Therefore, the statements 1. to 6., made above for groups $\mbox{$\mathcal{S}$}$, are valid for groups $\mbox{$\mathcal{P}$}$ as well, with the exception of statement 2. The latter is obvious: A macroscopic crystal is not periodic but `a massive block' of finite extension, and there is only one finite symmetry group $\mbox{$\mathcal{P}$}$ for the external shape of the crystal as compared to the infinite number of site-symmetry groups $\mbox{$\mathcal{S}$}$.

What is the essential difference between $\mbox{$\mathcal{S}$}$ and $\mbox{$\mathcal{P}$}$ ? Why can they not be identified ?

The description of the symmetry $\mbox{$\mathcal{P}$}$ is different from that of $\mbox{$\mathcal{S}$}$. The relation between $\mbox{$\mathcal{S}$}$ and the space group $\mbox{$\mathcal{R}$}$ is simple: $\mbox{$\mathcal{S}$}$ is a subgroup of $\mbox{$\mathcal{R}$}$. The relation between $\mbox{$\mathcal{P}$}$ and $\mbox{$\mathcal{R}$}$ is more complicated and rather different. This will become clear from the following example.

Example. There are not many compounds known whose symmetry consists of the identity, translations, and 2-fold rotations. The symbol of their space groups $\mbox{$\mathcal{R}$}_1$ is $P2$. Several omphacites (rock-forming pyroxene minerals), high-temperature Nb$_2$O$_5$, Cu$_2$In$_2$O$_5$, and a few more compounds are reported to belong to space-group type $P2$.

The compound Li$_2$SO$_4\cdot$ H$_2$O is the best pyroelectric non-ferroelectric substance which is known today. Its space group $\mbox{$\mathcal{R}$}_2$ is $P2_1$ with the identity, translations, and 2-fold screw rotations $2_1$. There are many compounds, e.g. sugars, with the same kinds of symmetry operations.

Consider the points of point space. With regard to space group $\mbox{$\mathcal{R}$}_1$, there are points with site symmetry 2, namely all points situated on one of the 2-fold rotation axes. However, with regard to space group $\mbox{$\mathcal{R}$}_2$ there is no point with site symmetry 2, because screw rotations have no fixed points. Nevertheless, the symmetry of the macroscopic crystal is that of (identity and) a 2-fold rotation in both cases. One can say, that $\mbox{$\mathcal{R}$}_1$ and $\mbox{$\mathcal{R}$}_2$ have point groups of the same type, but exhibit strong differences in their site-symmetry groups.

In order to understand this difference it is useful to consider the determination of $\mbox{$\mathcal{P}$}$. A natural crystal is mostly distorted: the growth velocities of its faces have been influenced by currents of the medium from which the crystal has grown (liquid, gas), or by obstacles which have prevented the development of the ideal shape. Therefore, the faces present at a macroscopic crystal are replaced by their face normals for the determination of the macroscopic symmetry. These face normals are vectors which are independent of the state of development of the faces. Then $\mbox{$\mathcal{P}$}$ is determined from the symmetry operations which map the bundle of face-normal vectors onto itself. Thus, the group $\mbox{$\mathcal{P}$}$ is a group of symmetry in vector space.

It is the conceptual difference between vector space and point space, experienced already in Section 1.4 when considering origin shifts, which leads to the difference between the groups $\mbox{$\mathcal{P}$}$ and $\mbox{$\mathcal{S}$}$. The symmetry operations of $\mbox{$\mathcal{S}$}$ are mappings of point space, whereas the symmetry operations of $\mbox{$\mathcal{P}$}$ are mappings of vector space. In Section 4.4 the description of these operations by matrices will be dealt with. It will turn out that the difference between $\mbox{$\mathcal{S}$}$ and $\mbox{$\mathcal{P}$}$ is reflected in the kinds of matrices which describe the operations of $\mbox{$\mathcal{S}$}$ and $\mbox{$\mathcal{P}$}$.

The above example of the space groups $P2$ and $P2_1$ has shown that there are space groups for which the groups $\mbox{$\mathcal{P}$}$ and $\mbox{$\mathcal{S}$}$ may have the same order, namely in $P2$. This is a special property which deserves a separate name.

Definition (D 3.4.3) A space group is called symmorphic if there are site-symmetry groups $\mbox{$\mathcal{S}$}$ which have the same order as the point group $\mbox{$\mathcal{P}$}$ of the space group.

In the non-symmorphic space group $P2_1$, there is no group $\mbox{$\mathcal{S}$}$ with the order 2 of $\mbox{$\mathcal{P}$}$.

Copyright © 2002 International Union of Crystallography