This is an archive copy of the IUCr web site dating from 2008. For current content please visit
https://www.iucr.org.
![[IUCr Home Page]](/iucr-top/logos/iucrhome.gif)
![[Commission Home Page]](/iucr-top/logos/cteach.gif)




Next: Special aspects of the matrix formalism
Up: The description of mappings by ...
Previous: The matrix-column pairs of crystallographic symmetry
The `General Position' in IT A
In IT A the set of all symmetry operations is listed for all space groups.
As we have seen, space groups are infinite groups, and there is an
infinite number of space groups for each space-group type, see
Section 3.4. How can such a listing be done at all ? In this section
the principles are dealt with which make the listings possible, as
well as the points of view which determine the actual listings.
- Consider the infinite number of space groups belonging to a
certain space-group type. If one refers each space group to its
conventional coordinate system, one obtains a set of
matrix-column pairs for each
space group but all these sets are identical. (This is one way
to classify the space groups into space-group types.)
Therefore, a listing is necessary only for each space-group type, but
not for each space group. This means 230 listings for the space
groups and 17 listings for the plane groups, and these listings
are indeed contained in IT A. In reality the number of space-group
listings is higher by about 20 % because there is sometimes more
than one conventional coordinate system: different settings, different
bases, or different origins, see IT A.
- No doubt, there is an infinite number of symmetry operations for
each space group. How can they be listed in a book of finite volume ?
Let W be a symmetry operation and (W,w) its
matrix-column pair. Because the conventional bases are always lattice
bases, (W,w) can be decomposed into a translation
 with integer coefficients and the matrix
with integer coefficients and the matrix
 :
:
 |
(4.6.1) |
For the coefficients
 holds.
By this decomposition one splits the infinite set of pairs
(W,w) into a finite set of representatives
holds.
By this decomposition one splits the infinite set of pairs
(W,w) into a finite set of representatives
 and an infinite set of translations
and an infinite set of translations
 . Clearly, only the representatives need to be
listed. Such a list is contained in IT A for each space-group type;
the number of necessary entries is reduced from infinite to at most 48.
. Clearly, only the representatives need to be
listed. Such a list is contained in IT A for each space-group type;
the number of necessary entries is reduced from infinite to at most 48.
For primitive bases, the list is complete and unique. There are
ambiguities for centered settings, see the remarks to definition
(D 1.5.2). For example, for a space group with an  -centered
lattice, to each point
-centered
lattice, to each point  there belongs a translationally
equivalent point
there belongs a translationally
equivalent point
 . Nevertheless, only one
entry
. Nevertheless, only one
entry
 is listed. Again, instead of
listing a translationally equivalent pair for each entry, the centering
translation is extracted from the list and written once for all on top
of the listing. For example,
the rational translations for the
is listed. Again, instead of
listing a translationally equivalent pair for each entry, the centering
translation is extracted from the list and written once for all on top
of the listing. For example,
the rational translations for the  -centered lattice are indicated
by `(0,0,0)+
-centered lattice are indicated
by `(0,0,0)+
 '.
For each of the matrix-column pairs
'.
For each of the matrix-column pairs
 ,
listed in the sequel, not only the products
,
listed in the sequel, not only the products
 have to be taken into account, but also
the products
have to be taken into account, but also
the products
 . (The term
. (The term
 is a symbol for
the column with all coefficients
is a symbol for
the column with all coefficients  .) The following example 3
(General position for space-group type
.) The following example 3
(General position for space-group type  , No. 199) provides such a listing.
, No. 199) provides such a listing.
- The representatives
 could be
listed as matrix-column pairs but
that would be wasting space. Although one could not save much space
with further conventions when listing general matrices, the simple
(48 orthogonal + 16 other = 64) standard matrices of crystallography
with their many zeroes have a great potential for rationalization.
Is there any point to list thousands of zeroes ? Therefore, in
crystallography an efficient procedure is applied to condense the
description of symmetry by matrix-column pairs considerably.
This method works like the shorthand notation for the normal
language, when the usual letters are replaced by shorthand symbols.
could be
listed as matrix-column pairs but
that would be wasting space. Although one could not save much space
with further conventions when listing general matrices, the simple
(48 orthogonal + 16 other = 64) standard matrices of crystallography
with their many zeroes have a great potential for rationalization.
Is there any point to list thousands of zeroes ? Therefore, in
crystallography an efficient procedure is applied to condense the
description of symmetry by matrix-column pairs considerably.
This method works like the shorthand notation for the normal
language, when the usual letters are replaced by shorthand symbols.
The equations (4.1.1) on p. ![[*]](crossref.gif) are shortened in the
following way:
are shortened in the
following way:
- The left side and the `=' sign are omitted
- On the right side, all terms with coefficients 0 are omitted
- Coefficients `+1' are omitted, coefficients `-1' are replaced
by `-' and are frequently written on top of the variable:
 instead of
instead of  , etc.
, etc.
- The three different rows are written in one line but separated
by commas.
3 examples shall display the procedure.
Example 1.

 .
.
The shorthand notation of IT A reads
 .
.
It is found in IT A under space group  , No. 96 on p. 367.
There it is entry (4) of the first block (the so-called General position)
under the heading
Positions.
, No. 96 on p. 367.
There it is entry (4) of the first block (the so-called General position)
under the heading
Positions.
Example 2.

is written in the shorthand notation of IT A
 ;
space group
;
space group  , No. 186
on p. 575 of IT A.
It is entry (5) of the General position.
, No. 186
on p. 575 of IT A.
It is entry (5) of the General position.
Example 3. The following table is the actual
listing of the General position for space-group type  , No. 199
in IT A on p. 603. The 12 entries, numbered (1) to (12), are to be taken
as they are (indicated by (0,0,0)+) and in addition with 1/2 added
to each element
, No. 199
in IT A on p. 603. The 12 entries, numbered (1) to (12), are to be taken
as they are (indicated by (0,0,0)+) and in addition with 1/2 added
to each element  (indicated by
(indicated by
 . Altogether these are 24 entries, which is announced
by the first number in the row, the `Multiplicity'. The reader is
recommended to convert some of the entries into matrix-column pairs or
. Altogether these are 24 entries, which is announced
by the first number in the row, the `Multiplicity'. The reader is
recommended to convert some of the entries into matrix-column pairs or
 matrices.
matrices.
|
Positions
Multiplicity,
Wyckoff letter,
Site symmetry
|
Coordinates
|
|

|
The listing of the `General position'
kills two birds with one stone:
- (i)
- each of the numbered entries lists the coordinates of an image
point
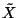 of the original point X under a symmetry operation
of the space group.
of the original point X under a symmetry operation
of the space group.
- (ii)
- Each of the numbered entries of the General position lists
a symmetry operation of the space group by the shorthand notation of
the matrix-column pair. This fact is not as obvious as the meaning
described under (i) but it is much more important. Knowing this way
one can extract and make available for calculations the full analytical
symmetry information of the space group from the tables of IT A.
Exactly one image point belongs to each of the infinitely many
symmetry operations and vice versa. Some of these points
are displayed in Figure 3.5.2 on p. ![[*]](crossref.gif) .
.
Definition (D 4.6.2) The set of all points which are symmetrically equivalent
to a starting point  (and thus to each other) under the symmetry
operations of a space group
(and thus to each other) under the symmetry
operations of a space group
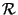 is called a point orbit
is called a point orbit
 of the space group.
of the space group.
Remarks.
- The starting point is a point of the orbit because it is mapped onto
itself by the identity operation (I,o) which is a
symmetry operation of any space group.
- The one-to-one correspondence between symmetry operations and
points is valid only for the General position, i.e. the first
block from top in the space-group tables. In this block the coordinate
triplets (shorthand symbols for symmetry operations) refer to points
which have site symmetry
 , i.e. only the identity
operation is a symmetry operation. In all the other blocks, the points
have site symmetries
, i.e. only the identity
operation is a symmetry operation. In all the other blocks, the points
have site symmetries
 with more than one site-symmetry
operation. If
with more than one site-symmetry
operation. If
 ,
also
,
also
 holds, where
holds, where
 is the order of
is the order of
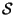 .
One can show, that the point
.
One can show, that the point  is mapped onto its image
is mapped onto its image 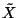 by
exactly as many symmetry operations of the space group
by
exactly as many symmetry operations of the space group
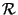 as is
the order of the site-symmetry group
as is
the order of the site-symmetry group
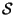 of
of  . Therefore, for
such points the symmetry operation can not be derived from the data listed
in IT A because it is not uniquely determined.
. Therefore, for
such points the symmetry operation can not be derived from the data listed
in IT A because it is not uniquely determined.
Definition (D 4.6.2) The blocks with points of site symmetries
 are called special positions.
are called special positions.
Different from the General position, a coordinate triplet of a special
position provides the coordinates
 of the image point of the starting point
of the image point of the starting point  only but no
information on a matrix-column pair.
only but no
information on a matrix-column pair.




Next: Special aspects of the matrix formalism
Up: The description of mappings by ...
Previous: The matrix-column pairs of crystallographic symmetry
Copyright © 2002 International Union of
Crystallography
IUCr
Webmaster
![]() -centered
lattice, to each point
-centered
lattice, to each point ![]() there belongs a translationally
equivalent point
there belongs a translationally
equivalent point
![]() . Nevertheless, only one
entry
. Nevertheless, only one
entry
![]() is listed. Again, instead of
listing a translationally equivalent pair for each entry, the centering
translation is extracted from the list and written once for all on top
of the listing. For example,
the rational translations for the
is listed. Again, instead of
listing a translationally equivalent pair for each entry, the centering
translation is extracted from the list and written once for all on top
of the listing. For example,
the rational translations for the ![]() -centered lattice are indicated
by `(0,0,0)+
-centered lattice are indicated
by `(0,0,0)+
![]() '.
For each of the matrix-column pairs
'.
For each of the matrix-column pairs
![]() ,
listed in the sequel, not only the products
,
listed in the sequel, not only the products
![]() have to be taken into account, but also
the products
have to be taken into account, but also
the products
![]() . (The term
. (The term
![]() is a symbol for
the column with all coefficients
is a symbol for
the column with all coefficients ![]() .) The following example 3
(General position for space-group type
.) The following example 3
(General position for space-group type ![]() , No. 199) provides such a listing.
, No. 199) provides such a listing.
![[*]](crossref.gif) are shortened in the
following way:
are shortened in the
following way:
 instead of
instead of  , etc.
, etc.
![[IUCr Home Page]](/iucr-top/logos/iucrhome.gif)
![[Commission Home Page]](/iucr-top/logos/cteach.gif)


