Scattering by a Classical Dipole Oscillator

In the classical theory of dispersion the atom is assumed to scatter radiation as if it was formed by dipole oscillators whose natural frequencies are those of the absorption edges of the electronic shells. These oscillators may be thought of as originated by simple harmonic vibrations of the electronic charges, as for instance, the movement of an electron of mass m around the positive nucleus assumed to be at rest. An electromagnetic wave falling on the atom and having an instantaneous electric field $\textbf {E} = \textbf {E}_0e^{i\omega t}$ at the position of the dipole, sets the electron in oscillation, the displacement of the atom satisfies then the differential equation:

$$\uml {\textbf x} + k{\textbf x} + \omega^2_s{\textbf x} = \frac{{eE}_{0}}{m}\cdot e^{i\omega t},$$ (1)

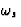where k is a damping factor and $\omega_s$ the natural circular frequency of the electron.

The forced solution of (1) is:

$${\textbf x} = \frac{{eE}_{0}}{m}\cdot \frac{e^{i\omega t}}{\omega^2_s - \omega^2 + ik \omega},$$ (2)

and the dipole moment:

$$M = e{\textbf x}$$ (3)

which has its axis in the direction of the applied field E.

This oscillating dipole radiates with the same frequency of oscillation; the amplitude of the wave at a unit distance in the equatorial plane being

$$A = \frac{e^2}{mc^2}\cdot \frac{\omega^2E_0}{\omega^2_s - \omega^2 + ik\omega}$$ (4)

The scattering factor of the dipole is defined, as usual, as the ratio of the scattered amplitude A to that scattered by a free electron A_e under the same conditions. In this case, A_e, the Thomson amplitude, is obtained by taking $\omega_s$ = 0, k = 0:

$$A_e = -\frac{e^2}{mc^2}\cdot E_0$$ (5)

The dipole scattering factor is then given by

$$f = \frac{A}{A_e} = \frac{\omega^2}{\omega^2 - \omega^2_s - ik\omega}$$ (6)

If the incident frequency $\omega$ tends to $\omega_s$ the scattering factor becomes imaginary.

Expression (6) is very important since the atomic scattering factor results from a superposition of similar terms by considering the atom as made up of a distribution of dipole oscillators. Let us denote by $f^{\prime}$($\omega$) and $f^{\prime\prime}$($\omega$) respectively the real and the imaginary parts of f:

$$f = f^{\prime} + if^{\prime\prime}$$ (7)

$$f^{\prime}(\omega) = \frac{\omega^2(\omega^2 - \omega^2_s)}{(\omega^2 - \omega^2_s)^2 + k^2\omega^2}$$

$$f^{\prime\prime}(\omega) = \frac{k\omega^3}{(\omega^2 - \omega^2_s)^2 + k^2\omega^2}$$

Apart from the functional dependence of f on the frequency, the main conclusion is that the scattering factor contains a real and an imaginary component, i.e. an in-phase and a quadrature component.

If a medium is composed of N similar dipoles per unit volume, it can be shown that the refractive index n is also complex and given by

$$n = 1 - \frac{2{\pi}Ne^2}{m\omega^2}\cdot f$$ (8)

which we rewrite as

$$n = 1 - \alpha - i\beta,$$

with

$$\alpha = \frac{2{\pi}Ne^2}{m\omega^2}\cdot f^{\prime},$$ (9)

$$\beta = \frac{2{\pi}Ne^2}{m\omega^2}\cdot f^{\prime\prime}.$$

The fact that n is complex and particularly so when $\omega$ approximates $\omega_s$, indicates that the medium is absorbent. In fact by taking the origin of phases at an arbitrary origin O, the phase, after the wave has travelled a distance r, is

$$e^{-i(2\pi/\lambda)nr} = e^{-i(2\pi/\lambda)r(1 - \alpha - i... ...{- (2\pi i\lambda)r(1 - \alpha)}\cdot e^{-(2\pi/\lambda)r\beta}$$

where the second factor is a real exponential with negative argument indicating a decrease in the wave amplitude. The decrease in intensity is given by $e^{- (4\pi/\lambda)r\beta}$ or $e^{-{\mu}r}$, $\mu$ being the linear absorption coefficient, then

$$\mu = \frac{4{\pi}\beta}{\lambda} = \frac{2\omega\beta}{c} = \frac{4{\pi}Ne^2}{mc\omega}\cdot f^{\prime\prime}(\omega)$$ (10)

After substitution of $f^{\prime\prime}$ and division by N one obtains the linear absorption coefficient per dipole in the medium for the circular frequency:

$$\mu_a(\omega) = \frac{4{\pi}e^2}{mc}\cdot \frac{k\omega^2}{(\omega^2 - \omega^2_s)^2 + k^2 \omega^2}.$$ (11)

Reciprocally, the imaginary component of the dipole scattering factor of an absorbent medium is given by:

$$f^{\prime\prime}(\omega) = \frac{mc}{4{\pi}e^2}\cdot {\omega}{\mu}_a(\omega).$$ (12)

If $\mu_a$ ($\omega$) was a measurable magnitude, the expression (12) would provide a way to calculate the imaginary component $f^{\prime\prime}$ by using the experimental values of the absorption coefficients. This conclusion, obtained for a dipole, is clearly true also for an atom, so that the tables of absorption coefficients can be regarded as giving the imaginary components of the atomic scattering factors except for a scaling coefficient.

Obviously $f^{\prime\prime}$ and $\mu_a$ have their respective maximum values for a frequency close to $\omega_s$; the value of the damping coefficient k being the breadth of the absorption peak at half height. The smaller the value of k the sharper the absorption peak which then becomes a line. If a beam of white radiation is passing through the medium only frequencies near to $\omega_s$ will be significantly absorbed.

Copyright © 1981, 1998 International Union of Crystallography