Next: The Oscillator Density Up: Anomalous Dispersion of X-rays in Crystallography Previous: Scattering by a Classical Dipole Oscillator
![[IUCr Home Page]](/iucr-top/logos/iucrhome.gif)
![[Commission Home Page]](/iucr-top/logos/cteach.gif)
![]()
![]()
![]()
Next: The Oscillator Density
Up: Anomalous Dispersion of X-rays in Crystallography
Previous: Scattering by a Classical Dipole Oscillator
Assuming the simple case where k is very small and ![]() is quite different
from
is quite different
from ![]() , then, we obtain approximately:
, then, we obtain approximately:
| (13) |
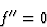 |
(14) |
|
|
Under these conditions, we shall concentrate on the real part ![]() of
the dipole scattering factor. Let us now consider an atom containing
g(1),
of
the dipole scattering factor. Let us now consider an atom containing
g(1),![]() , g(2),
, g(2),![]() , g(s),
, g(s),![]() dipole oscillators of
natural frequencies
dipole oscillators of
natural frequencies ![]() ,
,![]() ,
, ![]() ,
,![]() ,
,![]() ,
,![]() respectively. Expression (13) can now be generalized by summing the
contributions of all the oscillators contained in the atom so as to give
the real part of the atomic scattering factor:
respectively. Expression (13) can now be generalized by summing the
contributions of all the oscillators contained in the atom so as to give
the real part of the atomic scattering factor:
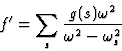 |
(15) |
The number g(s) of dipole oscillators of natural frequency ![]() existing in an atom is called the `oscillator strength' corresponding to that
particular frequency. The calculation of the oscillator strength is the main
difficulty in obtaining the resonance contributions to the atomic scattering
factors.
existing in an atom is called the `oscillator strength' corresponding to that
particular frequency. The calculation of the oscillator strength is the main
difficulty in obtaining the resonance contributions to the atomic scattering
factors.
We shall not discuss here either the implications or the validity of the generalization just made which has far reaching consequences. A comparison of these arguments with those from the quantum theory of atomic scattering is quite adequate, but will not be undertaken here, since we rather intend to concentrate on the applications of anomalous scattering. The reading is referred, for such a comparison, to James' book.61
If we now re-examine the foregoing arguments we note that the calculations were
explicitly made for points located in the plane perpendicular to the electric
vector of the incident wave, that is equivalent to taking the polarization
factor equal to unity. That is why the expression (15) obtained for the atomic
scattering factor is independent of the diffraction angle. In fact, as it is
well known, this is not generally true, for instance for a spherically symmetric
atom, f = f(sin ![]() /
/![]() ).
).
f could be independent of ![]() if the incident wavelength was big with
respect to the dimensions of the atom where the electron density is not
negligible; notwithstanding the opposite is the case normally encountered in
practice since the atomic dimensions and the wavelengths normally used in
crystallography are of the same order of magnitude, namely, one to two
ångströms.
if the incident wavelength was big with
respect to the dimensions of the atom where the electron density is not
negligible; notwithstanding the opposite is the case normally encountered in
practice since the atomic dimensions and the wavelengths normally used in
crystallography are of the same order of magnitude, namely, one to two
ångströms.
However, our aim is to obtain only the dispersion terms and not the entire
atomic scattering factor. The terms which correspond, for instance, to the K
absorption edge are important only when the incident frequency ![]() is close
to
is close
to ![]() . The relevant electron distribution in the case is only that of
the K electrons. It is then easy to verify that
. The relevant electron distribution in the case is only that of
the K electrons. It is then easy to verify that ![]() is much larger
than the dimensions of the atomic region where the K-electron density is
appreciable; this means that the phase difference in the scattered waves due to
the difference in position of the K electrons within the atom will be small
and their total contribution will thus be practically independent of the
diffusion angle. The same arguments are valid, mutatis mutandis , to the
case of L, M, etc., electrons. It follows that the resonant contribution to
the atomic scattering should be nearly independent of the diffusion angle, which
means that the hypothesis used in the previous calculations can be applied to a
fair approximation.
is much larger
than the dimensions of the atomic region where the K-electron density is
appreciable; this means that the phase difference in the scattered waves due to
the difference in position of the K electrons within the atom will be small
and their total contribution will thus be practically independent of the
diffusion angle. The same arguments are valid, mutatis mutandis , to the
case of L, M, etc., electrons. It follows that the resonant contribution to
the atomic scattering should be nearly independent of the diffusion angle, which
means that the hypothesis used in the previous calculations can be applied to a
fair approximation.
In the quantum mechanical treatment the analogues of the classical oscillator strengths are magnitudes g(k, n) which are proportional to the transition probability of an electron passing from a state k to a state n. For an electron atom,
| (16) |
Summing up, the atom scatters as if it was composed by dipole oscillators of given natural frequencies, identical to the Bohr frequencies, their number or oscillator strength being proportional to the transition probability of state k into state n. It is here important to note that the states k include all the discrete states of negative energy and the continuum of positive energy states.
Copyright © 1981, 1998 International Union of Crystallography
IUCr Webmaster