Next: References Up: Anomalous Dispersion of X-rays in Crystallography Previous: Use of the Photoelectric Absorption Measurements
![[IUCr Home Page]](/iucr-top/logos/iucrhome.gif)
![[Commission Home Page]](/iucr-top/logos/cteach.gif)
![]()
![]()
![]()
Next: References
Up: Anomalous Dispersion of X-rays in Crystallography
Previous: Use of the Photoelectric Absorption Measurements
The imaginary component of the atomic scattering factor corresponds to a
component of the scattered radiation from the atom having a phase in the forward
direction that lags ![]() /2 behind that of the primary wave.
/2 behind that of the primary wave.
As is known, in the (Thomson) scattering by free electrons, or very
approximately in the case of bound electrons and very high frequencies the
phase-lag of the forward scattered wave is ![]() . As the frequency of the
incident radiation approaches an absorption edge the amplitude of the imaginary
component increases so that the resultant phase may differ significantly from
. As the frequency of the
incident radiation approaches an absorption edge the amplitude of the imaginary
component increases so that the resultant phase may differ significantly from
![]() .
.
In order to have an appreciable resonant effect, the incident frequency must lie very close to one of the natural oscillator frequencies. However, since the virtual oscillators in an atom have frequencies covering a continuous distribution, the resonance effect may be appreciable even for frequencies not so close to one of the absorption edges, for it still coincides with one of the continuous distribution. A system having a discrete set of oscillators would then behave in an entirely different way.
Let us consider, for instance, the case of K electrons. As we know
![]() = 0 for
= 0 for ![]() <
< ![]() . It is
then only necessary to consider frequencies larger than
. It is
then only necessary to consider frequencies larger than ![]() , since only
in this case they may coincide with those of the continuum.
, since only
in this case they may coincide with those of the continuum.
An extension of equation (12) gives the relationship between the
K-contribution to the absorption coefficient and ![]() ,the K-contribution to the imaginary component of the atomic scattering factor:
,the K-contribution to the imaginary component of the atomic scattering factor:
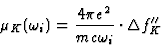
| (12') |
From relation (23) between the oscillator density and the absorption coefficient one obtains:
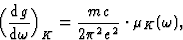
| (23') |
| (27) |
Assuming a dependence of ![]() on
on ![]() according to (24),
according to (24),
| (28) |
It is interesting to express the relationship between
![]() and the corresponding oscillator gK:
and the corresponding oscillator gK:
| (29) |
Figure 2 represents ![]() as a function of
as a function of
![]() /
/![]() , the effect of damping is indicated qualitatively
by the dotted line.
, the effect of damping is indicated qualitatively
by the dotted line.
The atomic scattering factor may be expressed as the sum of three terms:
![]()
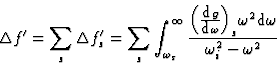 |
(30) |
| (31) |
![]()
It is possible by using quantum mechanical methods to determine the oscillator densities from the atomic wave functions. This method was first introduced by Hönl,62-63 who, of course, did not have at the time the computing facilities nowadays provided by modern computers, so that his main contribution was in developing the method and calculating some values for hydrogen-like K-electrons.
A method based on the utilization of the relationships between oscillator strengths and densities from one side and photoelectric absorption coefficients on the other, developed by Parratt and Hempstead,36 did not succeed initially in providing satisfactory results due mainly to the lack of good experimental values of the absorption coefficients and also, to my belief, to the lack of reliable experimental and theoretical atomic scattering factors.
Cromer67 used self-consistent field relativistic Dirac-Slater wave
functions to calculate accurate oscillator strengths for elements 10 through 98.
He calculated then the dispersion terms ![]() by using Parratt
and Hempstead's36 solution of equation (26) and summing over the different
absorption edges. The imaginary terms
by using Parratt
and Hempstead's36 solution of equation (26) and summing over the different
absorption edges. The imaginary terms ![]() were also computed
for the same elements from equation (29). The values of the parameters n used
by Cromer,67 Dauben and Templeton28 and other authors were taken from
the discussion by Parratt and Hempstead,36 as n = 11/4 for the 1s1/2
edge, n = 7/3 for the 2s1/2 edge and n = 5/2 for all other edges.
were also computed
for the same elements from equation (29). The values of the parameters n used
by Cromer,67 Dauben and Templeton28 and other authors were taken from
the discussion by Parratt and Hempstead,36 as n = 11/4 for the 1s1/2
edge, n = 7/3 for the 2s1/2 edge and n = 5/2 for all other edges.
The use of the dispersion terms for the solution of crystal structures, which
will be discussed later, made it necessary to obtain the values of
![]() for a wide range of wavelengths for atoms where the
anomalous dispersion effects are significant. This calculation was performed by
Saravia and Caticha-Ellis33 for elements 20 through 83 for 32 different
for a wide range of wavelengths for atoms where the
anomalous dispersion effects are significant. This calculation was performed by
Saravia and Caticha-Ellis33 for elements 20 through 83 for 32 different
![]() wavelengths ranging from Ti
wavelengths ranging from Ti![]() (2.75 Å) to
I
(2.75 Å) to
I![]() (0.435 Å), by using the
method of Parratt and Hempstead,36 the absorption edges from Cauchois and
Hulubei74 and the oscillator strengths from Cromer.67 Essentially the
same calculations were later performed by Hazell34 for eleven
(0.435 Å), by using the
method of Parratt and Hempstead,36 the absorption edges from Cauchois and
Hulubei74 and the oscillator strengths from Cromer.67 Essentially the
same calculations were later performed by Hazell34 for eleven ![]() radiations.
radiations.
Copyright © 1981, 1998 International Union of Crystallography
IUCr Webmaster