Large |E_H|, |E_K| and |E_-H-K|: The Triplet Relationship

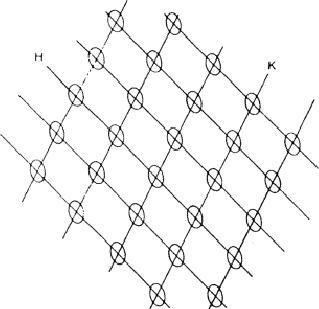
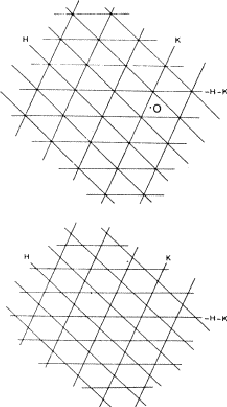
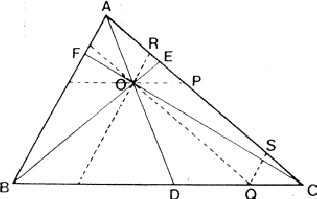
If two reflections H and K are both strong then the electron density is likely to be found in the neighbourhood of the two sets of equidistant planes defined by H and K. That is to say the electron density will be found near the lines of intersection of the planes H and K as indicated in projection in Fig. 9. A large |E| for reflection -H-K as well implies that the electron density will also peak in planes lying d_-H-K apart. It is therefore most likely that these planes run through the lines of intersection of the planes H and K, in other words that the three sets of planes have their lines of intersection in common (see Fig. 10a). Then by choosing an origin at an arbitrary point the triplet phase relationship can be found from a planimetric theorem, proved in Fig. 11:

AO/AD + BO/BE + CO/CF = 2

which is equivalent to

$$\phi_{-H-K} + \phi_H + \phi_K + 2 {\cdot} 2 {\pi} = 0\quad(\mbox{modulo } 2 {\pi}).$$ (15)

Because the choice of the origin is arbitrary it is obvious that expression (15) is independent of the position of the origin: relations of this type are usually called `structure invariants', although a more logical name would be `origin invariants'.

Figure 8: A few large terms (I: F_KF_H-K; II: F_K'F_H-K'; etc) from the right hand side of expression (27) in a phase diagram. It can be seen that their phases (1: $\phi_K + \phi_{H-K}$; 2: $\phi_{K'} + \phi_{H-K'}$; etc) are approximately equal to $\phi_H$.

Figure 9: If the reflections H and K are both strong, then the electron density will likely lie in the neighbourhood of the intersecting lines of the two sets of equidistant planes defined by H and K.

Figure 10: When H and K are strong and -H-K is strong as well it is more likely that the planes of high density of -H-K run through the lines of intersection (a) than just in betwen (b).

Figure 11: In an arbitrary triangle ABC an origin O has been arbitrarily drawn. Theorem: AO/AD + BO/BE + CO/CF = 2. Proof: AO/AD = AP/AC; CO/CF = CR/AC; BO/BE = BQ/BC = AS/AC; because RP = SC, AP + CR +AS = 2AC.

In Fig. 10a the ideal situation is sketched and of course a small shift of the planes of largest density of -H-K does not affect the reasoning given above. However, the most unlikely position for these planes is the one indicated in Fig. 10b; here the planes -H-K of largest electron density keep clear of the lines of intersection of H and K. The triplet relationship therefore has a probability character and this is emphasised by formulating it as

$$\phi_H + \phi_K + \phi_{-H-K} \approx 0$$ (16)

for large values of E₃ = N^-1/2|E_HE_KE_-H-K|. The $\approx$-sign means that the most probable value of the triplet phase sum is 0. Clearly, the triplet product E₃ is large when all three reflections H, K and -H-K have large |E|-values.

Copyright © 1984, 1998 International Union of Crystallography