This is an archive copy of the IUCr web site dating from 2008. For current content please visit
https://www.iucr.org.
![[IUCr Home Page]](/iucr-top/logos/iucrhome.gif)
![[Commission Home Page]](/iucr-top/logos/cteach.gif)




Next: Crystallographic site-symmetry operations
Up: Crystallographic symmetry
Previous: Crystallographic symmetry
Isometries
An isometry W, see also Section 2.1,
- maps each point P of the point space onto exactly one image
point
 :
:
 ;
;
- is a mapping of the point space onto itself which leaves all
distances and thus all angles invariant.
There are different types of isometries which will be characterized in
this section. For this characterization the notion of fixed points
is essential.
Definition (D 3.1.1) Let W be an isometry and P a point of
space. Then P is called a fixed point of the isometry
W if it is mapped onto itself (another term: is
left invariant) by W, i.e. if the image point
 is equal to the original point
is equal to the original point 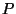 :
:
 .
.
The isometries are classified by their fixed points, and the fixed points
are often used to characterize the isometries in visual geometric terms,
see the following types of isometries. Besides the `proper' fixed points
there are further objects which are not fixed or left invariant pointwise
but only as a whole. Lines and planes of this kind are of great interest
in crystallography, see the following examples.
Kinds of isometries.
The kinds 1. to 4. of isometries in the following list
preserve the so-called `handedness' of the objects: if a right (left)
glove is mapped by one of these isometries, then the image is also a
right (left) glove of equal size and shape. Such isometries are also
called isometries of the first kind
or proper isometries.
The kinds 5. to 8. change the `handedness': the image of
a right glove is a left one, of a left glove is a right one. These kinds
of isometries are often called isometries of the second
kind or improper isometries.
- Identity I. The identity
mapping maps each point onto itself, each point of space is a fixed point.
All lines and planes of the space are left invariant as well.
- Translation T. By a
translation each point of the point space is shifted in the same
direction by the same amount, such that the translation vector
r from each original point P
to its image point
 is independent of the point P. There is no proper
fixed point. Nevertheless, each line L parallel to r
is mapped onto itself as a whole, as is each plane which contains
L. [The identity mapping may be considered as a special
translation with r = o, where o is the zero
vector of length zero, see Section 1.3. Except if it is mentioned
explicitly, the term `translation' is used for proper translations
only, i.e. for translations with
is independent of the point P. There is no proper
fixed point. Nevertheless, each line L parallel to r
is mapped onto itself as a whole, as is each plane which contains
L. [The identity mapping may be considered as a special
translation with r = o, where o is the zero
vector of length zero, see Section 1.3. Except if it is mentioned
explicitly, the term `translation' is used for proper translations
only, i.e. for translations with
 .]
.]
- Rotation. Each rotation maps a
line of points onto itself pointwise. This line is called the
rotation axis. The whole space is
rotated around this axis by an angle
 , the rotation angle.
The unit vector
, the rotation angle.
The unit vector
 parallel to the rotation axis is
called the direction of the rotation axis. Each plane perpendicular
to the rotation axis is mapped onto itself as a whole: it is rotated about
the intersection point of the plane with the rotation axis. For a 2-fold
rotation also each plane containing the rotation axis is left invariant
as a whole. [The identity operation may be considered as a
special rotation with the rotation angle
parallel to the rotation axis is
called the direction of the rotation axis. Each plane perpendicular
to the rotation axis is mapped onto itself as a whole: it is rotated about
the intersection point of the plane with the rotation axis. For a 2-fold
rotation also each plane containing the rotation axis is left invariant
as a whole. [The identity operation may be considered as a
special rotation with the rotation angle
 . Except if it
is mentioned explicitly, the term `rotation' is used for proper
rotations only, i.e. for rotations with
. Except if it
is mentioned explicitly, the term `rotation' is used for proper
rotations only, i.e. for rotations with
 .]
.]
- Screw rotation. A screw
rotation is a combination of a rotation (
 is the
direction of the rotation axis) and a translation with its translation
vector parallel to u
is the
direction of the rotation axis) and a translation with its translation
vector parallel to u . A screw rotation leaves no
point fixed, the rotation axis of the involved rotation is called
the screw axis, and the vector of the involved translation
is the screw vector. The screw axis is not left fixed
pointwise but as a whole only (it is shifted parallel to itself
by the involved translation). In general the result of the combination
of 2 isometries depends on the sequence in which the isometries
are performed. The screw rotation, however, is independent of the
sequence of its 2 components.
. A screw rotation leaves no
point fixed, the rotation axis of the involved rotation is called
the screw axis, and the vector of the involved translation
is the screw vector. The screw axis is not left fixed
pointwise but as a whole only (it is shifted parallel to itself
by the involved translation). In general the result of the combination
of 2 isometries depends on the sequence in which the isometries
are performed. The screw rotation, however, is independent of the
sequence of its 2 components.
- Inversion. An inversion is the
reflection of the whole space in a point
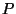 , which is called the
center of inversion. The point
, which is called the
center of inversion. The point 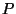 is the only fixed point. Each line or plane through
is the only fixed point. Each line or plane through 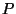 is mapped onto
itself as a whole because it is reflected in
is mapped onto
itself as a whole because it is reflected in 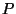 . The inversion is an
isometry of the second kind: any right glove is mapped onto a left one
and vice versa.
. The inversion is an
isometry of the second kind: any right glove is mapped onto a left one
and vice versa.
- Rotoinversion. A
rotoinversion can be understood as a combination of a rotation with
 and
and
 and
an inversion, where the center of inversion is placed on the rotation
axis of the rotation. A rotoinversion is an isometry of the second
kind. The inversion point (which is no longer a center of inversion !)
is the only fixed point; the axis of the rotation, now called
rotoinversion axis, is the only
line mapped onto itself as a whole, and the plane through the inversion
point and perpendicular to the rotoinversion axis is the only
plane mapped onto itself as a whole. Again, a rotoinversion does not
depend on the sequence in which its components are performed.
and
an inversion, where the center of inversion is placed on the rotation
axis of the rotation. A rotoinversion is an isometry of the second
kind. The inversion point (which is no longer a center of inversion !)
is the only fixed point; the axis of the rotation, now called
rotoinversion axis, is the only
line mapped onto itself as a whole, and the plane through the inversion
point and perpendicular to the rotoinversion axis is the only
plane mapped onto itself as a whole. Again, a rotoinversion does not
depend on the sequence in which its components are performed.
- Reflection. A reflection is
another isometry of the second kind. Each point of space is reflected
in a plane, the reflection plane or mirror plane,
such that all points of this plane, and only these points, are fixed
points. In addition, each line and each plane perpendicular to the
mirror plane is left invariant as a whole.
- Glide reflection. A glide reflection
is an isometry of the second kind as well.
It can be conceived as a combination of a reflection in a plane and
a translation parallel to this plane. The mirror plane of the
reflection is now called a
glide plane. The translation vector of the translation involved
is called the glide vector g. There is no fixed point
of a glide reflection. Left invariant as a whole are the glide plane and
those planes which are perpendicular to the glide plane and parallel to
g as well as those lines of the glide plane which are parallel
to g.
Crystallographic symmetry operations may belong to any of these kinds of
isometries. They are designated in text and formulae by the so-called
Hermann-Mauguin symbols and in drawings by specific symbols which are
all listed in IT A, Section 1 as well as in the Brief Teaching Edition of
Vol. A. Although each kind of isometries is represented among the
crystallographic symmetry operations, there are restrictions which will
be dealt with in the next 2 sections.




Next: Crystallographic site-symmetry operations
Up: Crystallographic symmetry
Previous: Crystallographic symmetry
Copyright © 2002 International Union of
Crystallography
IUCr
Webmaster
![[IUCr Home Page]](/iucr-top/logos/iucrhome.gif)
![[Commission Home Page]](/iucr-top/logos/cteach.gif)
![]() is equal to the original point
is equal to the original point ![]() :
:
![]() .
.