The Oscillator Density

For the continuum of positive energy states, the summations become integrals. The natural frequency for them changes continuously, so that rather than the discrete value g($\omega_s$) it is necessary to define the oscillator density (dg/d$\omega$) at the frequency $\omega$. The number of oscillators with frequencies between $\omega$ and $\omega$ + d$\omega$ is (dg/d$\omega$)d$\omega$. This number is zero for $\omega$ < $\omega_s$, where $\omega_s$ is the frequency associated with the s absorption edge. The oscillator strength due to all K electrons for instance, is obtained by integration in the whole range of frequencies $\omega_K$ to $\infty$, i.e. the interval where the number of oscillators related to the continuum of positive energy states is different from zero:

$$g_K = \int^{\infty}_{{\omega}_K} \Big(\frac{\mbox{d}g}{\mbox{d}\omega}\Big)_K \mbox{d}\omega.$$ (17)

In words, g_K is given by the probability of transition of the K electrons to all permissible states. Wheeler and Bearden⁶² applied the sum rule and obtained

$$g_K = 2\Big(1 - \sum_m g(k, m)\Big)$$ (18)

where g(k, m) is the oscillator strength of the virtual oscillator of the transition $k{\rightarrow}m$, where m is an occupied state. The sum in (18) then needs be taken only on the relatively few occupied states. g_K is then less than 2. Analogous considerations apply to the other shells, so that for an s shell one would have:

$$g_s = n_s \Big(1 - \sum_m g(s, m)\Big)$$ (19)

when n_s is the multiplicity of the shell. This method of calculation applied by Wheeler and Bearden in 1934 to the K-electrons of a few atoms has apparently some advantages which have not been exploited again.

Bethe⁶⁵ calculated the oscillator strengths g(s, m) for hydrogen-like atoms.

From equation (19), the generalized Thomas-Reiche-Kuhn rule can be obtained observing that: g(s, m) = -g(m, s). The justification of this argument is of a statistical nature, for both transitions should have the same probability, since their net result must average zero.

Thus,

$$\sum_s g(s) = \sum_s \int^{\infty}_{{\omega}_s} \Big(\frac{\mbox{d}g}{\mbox{d}\omega}\Big)\mbox{d}\omega = Z$$ (20)

$$s = K, L, M, \dots$$

Equation (15), which can be rewritten:

$$f^{\prime} = \sum_s g(s) - \sum_s \frac{g(s){\omega}^2_s}{{\omega}^2_s - {\omega}^2},$$

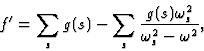
(15')

becomes

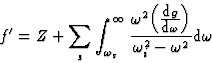$$f^{\prime} = Z + \sum_s \int^{\infty}_{{\omega}_s} \frac{{\o... ...}{\mbox{d}\omega}\Big)}{{\omega}^2_i-{\omega}^2} \mbox{d}\omega$$ (21)

$$s = K, L, M, \dots$$

Equation (21) only applies when $\omega_i$, the incident frequency, corresponds to a wavelength ${\lambda}_i$ large in comparison with the atomic dimensions. For frequencies higher than the natural frequencies of the atom and wavelengths of the order of the atomic dimensions, we may substitute Z by f₀, the normal scattering factor, with good approximation and write:

$$f^{\prime} = f_0 + \Delta f^{\prime}$$

where

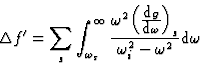$${\Delta}f^{\prime} = \sum_s \int^{\infty}_{{\omega}_s} \frac... ...\mbox{d}\omega}\Big)}_s}{{\omega}^2_i-{\omega}^2}\mbox{d}\omega$$ (22)

Equations (21) and (22) are valid for any wavelength except for very short ones when relativistic corrections are not negligible. Damping has also been neglected: an approximation usually adopted in the calculation of ${\Delta}f^{\prime}$ and ${\Delta}f^{\prime\prime}$, which however does not introduce unduly large errors except in the intervals

$$x = \frac{{\omega}_i}{{\omega}_s} \cong 1 \pm 0.005,$$

around the absorption edges.

The `normal' scattering factor f₀ has Z as a limiting value at low frequencies for any angle of scattering and at very low angles for any frequency. To obtain the real part of the dispersion correction one has to integrate equation (22) so that the values of the oscillator densities (dg/d$\omega$) have to be calculated. This can be done from the atomic wave-functions. Hönl^62-63 made calculations for the K and L electrons which were assumed to be hydrogen-like. The result was quite satisfactory for the K electrons but not for the L's. Later Hönl's method was applied by Eisenlohr and Müller⁶⁶ to the L electrons of several atoms.

The Hartree wave functions were tried by Cromer⁶⁷ to obtain the oscillator strengths, but he found them inadequate for this purpose, particularly for the heavy elements. He tried then the relativistic wave functions without exchange, calculated by Cohen⁶⁸ in the test cases of tungsten and uranium, finding better results. New relativistic wave functions computed by Lieberman, Waber and Cromer⁶⁹ became available for all atoms, which included Slater's^{70- 71} approximate exchange correction and Latter's⁷² self-interaction term. Using these wave functions, Cromer⁶⁷ calculated a set of oscillator strengths which have been in use up to now. From them, he obtained a set of dispersion corrections for elements 10 through 98 for five different wavelengths.

Copyright © 1981, 1998 International Union of Crystallography