Discussion List Archives

[Date Prev][Date Next][Thread Prev][Thread Next][Date Index][Thread Index]

Re: Ambiguity in atom_site.disorder_group value -1

Sure -- mention of "software" is irrelevant here. Totally agree there.

But still pondering this...

On Mon, Oct 17, 2022 at 5:18 PM Bollinger, John C via coreDMG <coredmg@iucr.org> wrote:
Hi Bob,

Yes, when a moiety is disordered about a symmetry element with multiplicity 2, such as a 2-fold rotation axis, there will be half as many per unit cell as there would ordinarily be for a moiety at a general position of the space group -- much the same as if it were ordered (and therefore symmetric) around the symmetry element.  Analogous applies for disorder around higher-symmetry positions.

My thinking is that the crystallographer might recognize "a" special symmetry element, but the application of symmetry creates n copies, not two, in a space group with n symmetry operations. Right?

Thus, fundamentally, there is no such concept of a "specific" symmetry element. All n copies symmetry related, but all are not "complementary".

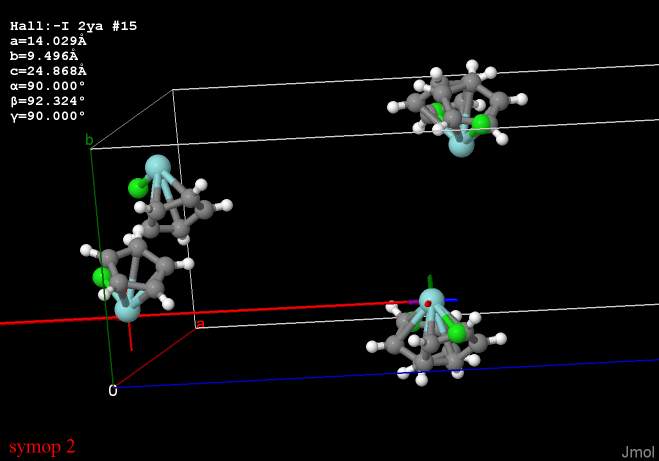
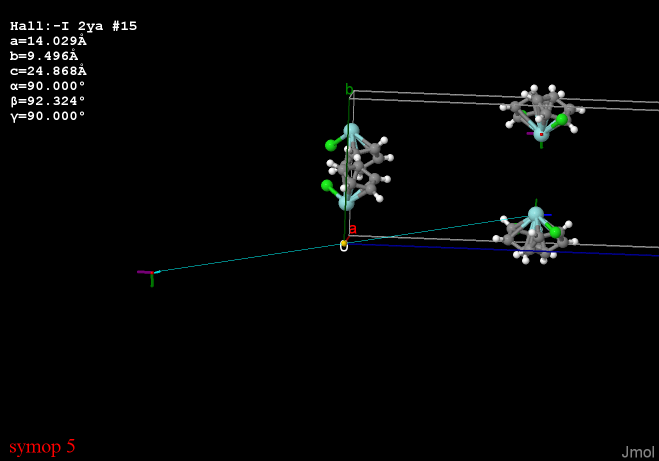
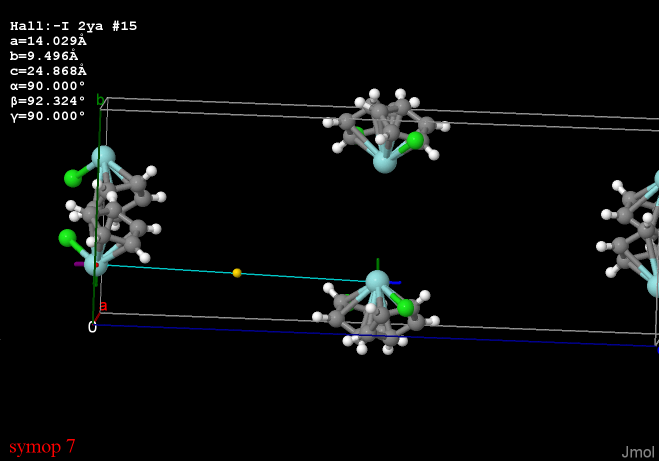
John, I appreciate the addition of "one or more" here. In the example I have, symmetry element 2 is a C2 axis parallel to the b axis in the ab plane that generates the overlapping pairs. Thus:

image.png

Symmetry operation 5 is a center of inversion at {0 0 0} that equally well describes the disorder, pairing them differently:
image.png

and symmetry element 7 is a center of inversion at {1/4, 1/4, 1/4}
image.png


This group can be considered to be disordered about any of these symmetry elements.

I'm not sure I understand this statement:

In addition to not being occupied simultaneously with sites belonging to different groups, the sites of such a group also are not occupied simultaneously with symmetry equivalents of sites belonging to the same group.  That is, the group's symmetry equivalents are among its disorder complements. 

I don't understand this statement. Why would it be true that sites related by symmetry are "not being occupied simultaneously"? If there are eight symmetry operations, four of those have to map to one disorder complement, and four have to map to the opposite. Pretty sure that has to be true, because we generated all eight from the same atoms. Don't half of those have to be occupied simultaneously?

True, only half of these can be occupied in any given unit cell, but I don't think we can know which of these four are occupied and which not in a given unit cell. Can we? -- Except for the obvious that if they have overlapping electron densities, then that's not possible.

To say it another way, I can pick any two Cl atoms in this unit cell and determine the space group operation relating them. Half of these, I guess, would be considered "complementary" but the others would be simultaneously occupied.

Do we even know if, in any given unit cell, half of the sites are of one complement and half are of the other? Or is it that we know that on average this must be true? How does that work?

I think only half of the group's symmetry equivalents are among its disorder complements. At least in this case. Pretty sure that is not a general statement, either.

Bob

_______________________________________________
coreDMG mailing list
coreDMG@iucr.org
http://mailman.iucr.org/cgi-bin/mailman/listinfo/coredmg

[Send comment to list secretary]
[Reply to list (subscribers only)]