Acta Cryst. (1984). A40, 399-404![]()
Nomenclature of polytype structures
Report of the International Union of Crystallography Ad-Hoc Committee on the nomenclature of disordered, modulated and polytype structures*
By A. GUINIER (Chairman), Université de Paris-Sud, Centre d'Orsay, Laboratoire de Physique des Solides, Bâtiment 510, 91405 Orsay, France
G. B. BOKIJ, Institute of Geology, Mineralogy and Petrography, Academy of Sciences of the USSR, Staromonetny 35, Moscow 109017, USSR
K. BOLL-DORNBERGER,J. M. COWLEY, Department of Physics, Arizona State University, Tempe, AZ 85287, USA
S. DUROVIC, Laboratory for Structure Research, Institute of Inorganic Chemistry, Slovak Academy of Sciences, Dúbravská cesta, 84236 Bratislava, Czechoslovakia
H JAGODZINSKI, Institut für Kristallographie und Mineralogie, Universität, Theresienstrasse 41, D-8000 München 2, Federal Republic of Germany
P. KRISHNA, Department of Physics, Banaras Hindu University, Varanasi-221005, India
P. M. DE WOLFF, Department of Technical Physics, Delft University of Technology, Lorentzweg 1, 2628 Delft, The Netherlands
B. B. ZVYAGIN, Institute of Geology, Mineralogy and Petrography, Academy of Sciences of the USSR, Staromonetny 35, Moscow 109017, USSR
D. E. COX, (ex officio, IUCr Commission on Neutron Diffraction), Physics Department, Brookhaven National Laboratory, Upton, NY 11973, USA
P. GOODMAN, (ex officio, IUCr Commission on Electron Diffraction), CSIRO Division of Chemical Physics, PO Box 160, Clayton, Victoria 3168, Australia
TH. HAHN, (ex officio, IUCr Commission on International Tables), Institut für Kristallographie, RWTH, Templergraben 55, D-5100 Aachen, Federal Republic of Germany
K. KUCHITSU, (ex officio, IUCr Commission on Electron Diffraction), Department of Chemistry, Faculty of Science, University of Tokyo, 3-1 Hongo 7-chome, Bunkyo-ku, Tokyo 113, Japan
and S. C. ABRAHAMS (ex officio, IUCr Commission on Crystallographic Nomenclature), AT&T, Bell Laboratories, Murray Hill, NJ 07974, USA
(Received 28 September 1983; accepted 25 January 1984)
* Appointed in 1979 by the IUCr Executive Committee under ground rules outlined in Acta Cryst . (1979). A35, 1072. Final Report accepted in 1983 by the IUCr Commission on Crystallographic Nomenclature and the Executive Committee.
![]() The major contributions made by K. Boll-Dornberger to this Report until her death in July 1981 are deeply appreciated.
The major contributions made by K. Boll-Dornberger to this Report until her death in July 1981 are deeply appreciated.
Abstract
An earlier report [Acta Cryst. (1977), A33, 681-684] by a joint IUCr-IMA committee on the nomenclature of polytypism has been revised and extended. Two kinds of symbolism are recommended for use with either simple or complicated polytypic structures. The first consists of 'indicative symbols' in a modified Gard notation, the second of 'descriptive symbols' based on earlier proposals by Dornberger-Schiff, Durovic and Zvyagin. The polytypism of ZnS and SrGeO3 provides examples for the use of descriptive symbolism.
Introduction
This report is concerned exclusively with the nomenclature of polytype structures. An earlier recommendation on polytype nomenclature was published in Acta Cryst. (1977), A33, 681-684 by a joint IUCr-IMA committee. In part III of that recommendation, a new symbolism that was based on the work of Dornberger-Schiff & Durovic (1974) and Zvyagin (1967, 1974) was proposed but not given in detail. The present report gives a full description of these symbols in §II.2 as approved by the Ad-Hoc Committee. For completeness, the basic 1977 recommendations are included in §§I and II.1 in modified form.
I. Definition
An element or compound is polytypic if it occurs in several different structural modifications, each of which may be regarded as built up by stacking layers of (nearly) identical structure and composition, and if the modifications differ only in their stacking sequence. Polytypism is a special case of polymorphism: the two-dimensional translations within the layers are (essentially) preserved whereas the lattice spacings normal to the layers vary between polytypes and are indicative of the stacking period. No such restrictions apply to polymorphism.
Comment : The above definition is designed to be sufficiently general to make polytypism a useful concept. There is increasing evidence that some polytypic structures are characterized either by small deviations from stoichiometry or by small amounts of impurities. (In the case of certain minerals like clays, micas and ferrites, deviations in composition up to 0.25 atoms per formula unit are permitted within the same polytypic series: two layer structures that differ by more than this amount should not be called polytypic.) Likewise, layers in different polytypic structures may exhibit slight structural differences and may not be isomorphic in the strict crystallographic sense.
The Ad-Hoc Committee is aware that the definition of polytypism above is probably too wide since it includes, for example, the turbostratic form of graphite as well as mixed-layer phyllosilicates. However, the sequence and stacking of layers in a polytype are always subject to well-defined limitations. On the other hand, a more general definition of polytypism that includes 'rod' and 'block' polytypes may become necessary in the future.
General recommendations concerning names commonly used in the studies of polytypic materials are given in the 1977 report.
II. Polytype symbolism
The Ad-Hoc Committee has examined the systems of structural symbols for polytypic structures already in the literature, which may be divided into two categories:
the first, of indicative symbols , includes systems that can be used without knowledge of the crystal structure; these necessarily make use of observable characteristics such as symmetry, periodicity of layers, interchange of axes, etc.;
the second, of descriptive symbols, includes systems that specify the exact stacking sequence of layers. Such systems allow the reader to deduce the unit cell and space group, and to recognize identical structures described by different axes or unit cells (settings); they facilitate comparison of structural data referring to different polytypes and allow identification by means of calculated intensities.
Most notational systems in the literature have been designed specifically for certain types of structure and, in the opinion of the Ad-Hoc Committee, cannot be applied universally. Several systems that showed promise were examined in more detail and have been further modified.
The Ad-Hoc Committee approves the system recommended in 1977 for the first category, see §II.1, and recommends the system described in §II.2 for the second category.
II.1. Indicative symbols: modified Gard notation
Gard (1966) has described a nomenclature for fibrous calcium silicates that was modified by A. Kato and H. Schulz in the 1977 report. The modified Gard notation is recommended here because it takes into account multiple translation periods along all three axes as well as the interchange of axes relative to a standard subcell. For polytypes without interchange of axes and with multiple periodicity normal only to the layers, a simplified version of the notation may be used which is similar to that introduced by Ramsdell (1947) for SiC and is in common use for phyllosilicates. Although the modified Gard notation has been adapted here to describe polytype structures, it is evident from the examples considered below that it can also be useful in nonpolytypic cases involving permutations of axes or superstructures in one or more directions.
In this system the symbol to describe a polytypic phase consists of a mineral name and an italicized supplement, combined by a hyphen. The supplement contains four positions, with additional marks when necessary. The first position in the symbol is filled by one of the following capital letters to indicate the crystal system of the compound:![[*]](https://www.iucr.org/__data/assets/image/0012/12621/foot_motif.gif)
| cubic | = C |
| hexagonal | = H |
| trigonal (with hexagonal Bravais lattice) | = T |
| trigonal (with rhombohedral Bravais lattice) | = R |
| tetragonal (quadratic) | = Q |
| orthorhombic | = O |
| monoclinic | = M |
| triclinic (anorthic) | = A. |
Three lower-case letters, accompanied by numbers when necessary, follow the symmetry symbol to indicate the periodicities along the three axes (in the order a, b, c ) of the compound relative to those of the smallest subcell of the polytypic system. Thus, molybdenite-2H is written as molybdenite-Haa2c and molybdenite-3R as molybdenite-Raa3c. In cases where the pseudosymmetry is of special interest, the letter ![]() (abbreviation of pseudo-) is added before the symmetry symbol. For example,
(abbreviation of pseudo-) is added before the symmetry symbol. For example, ![]() means pseudo-hexagonal. Thus, pearceite is written as pearceite-
means pseudo-hexagonal. Thus, pearceite is written as pearceite-![]() and arseno-polybasite as pearceite-
and arseno-polybasite as pearceite-![]() . The permutation of axes relative to that of the parent-structure subcell is easily indicated by permuting the appropriate symbols, for example (see Appendix 1), gibbsite-
. The permutation of axes relative to that of the parent-structure subcell is easily indicated by permuting the appropriate symbols, for example (see Appendix 1), gibbsite-![]() and gibbsite-
and gibbsite- ![]() . A definition of these species has been given by Strunz (1966).
. A definition of these species has been given by Strunz (1966).
In order to distinguish the ordered and disordered polytypes of xonotlite, described by Gard (1966) as P121 and ![]() 21, both of which are Ma2bc or
21, both of which are Ma2bc or ![]() in the proposed system, an additional symbol d (abbreviation for disordered) is added as a subscript to the letter involved. That is,
in the proposed system, an additional symbol d (abbreviation for disordered) is added as a subscript to the letter involved. That is, ![]() 21 is written as Mad2bc or
21 is written as Mad2bc or ![]() .
.
In order to reconcile the present notation with that of Ramsdell, the following simplification may be made. If the four-position symbol describes a tetragonal or hexagonal compound, then the first two axes have the same length, as in graphite-Haa2c. In this case, it is permissible to delete the first two axes and place the periodicity of the third axis in front of the capital letter symbolizing the crystal system. Thus graphite-Haa2c becomes graphite-2H. This rule is also applicable to polytypes with pseudo-hexagonal symmetry. In this case, the deleted letters are not always aa but may be bc, ab, ba, etc., where ![]() 3. Thus, indialite may be given as cordierite-1H and cordierite (sensu stricto ) as cordierite-1O or cordierite- 1
3. Thus, indialite may be given as cordierite-1H and cordierite (sensu stricto ) as cordierite-1O or cordierite- 1![]() .
.
In the case of the polytypic micas, 2M1 and 2M2 require different treatment. Thus, muscovite-2M1 = muscovite-Mab2c or -![]() can be shortened to muscovite-2M or -2
can be shortened to muscovite-2M or -2![]() . But for muscovite-2M2 = muscovite-Mba2c or -
. But for muscovite-2M2 = muscovite-Mba2c or -![]() , the long symbol should be retained if the reversal of two axes is to be described. This system, however, is not intended to replace symbols such as 2M1 and 2M2 that are useful and internationally accepted.
, the long symbol should be retained if the reversal of two axes is to be described. This system, however, is not intended to replace symbols such as 2M1 and 2M2 that are useful and internationally accepted.
Examples of the recommended nomenclature are given in Appendix 1 for a representative number of mineral groups. The mineral names tabulated are chosen for familiarity and are intended to be illustrative, rather than exhaustive, and cover related phases by the least number of names. The Ad-Hoc Committee does not necessarily recommend that all traditional names be dropped. Polytypic non-minerals are to be referred to by their chemical formula and appropriate supplement.
II.2. Descriptive symbols: notation
In certain polytypic compounds a number of different polytypic structures may have an identical modified Gard symbol. A more detailed symbolism is hence desirable to allow specification of the exact stacking sequence of layers, if known. Such detailed symbols are necessarily complex, and the ideal goal is a symbol that gives the necessary information in a readily understandable format. Too complex a symbol will probably be used only by a few experts in the field.
In the present recommendation, the stacking sequence of two-dimensionally periodic building layers (BLs) in any polytype is described by a sequence of conventionally chosen characters (numbers, letters, signs, etc. ) which indicate the absolute orientation of each BL and the relative displacements of their origins. Before presenting the symbols for a given polytypic family, the choice of BLs, their origins, possible orientations and stacking possibilities are to be specified - e.g. by indicating the orientational and displacement vectors in diagrams of the normal projection of the structure onto the plane parallel to the BLs relative to a fixed coordinate system. The choice of orientational and displacement characters and other relevant items should also be given in this explanatory introduction .
The orientational characters should be in upper-case and the displacement characters in lower-case letters (denoting different kinds of BLs and structural displacements, respectively): both may have subscripts [integer numbers, sign (+, -), etc .], chosen by convention, to indicate respective orientation and displacement. The sequence of BLs is hence given by a corresponding sequence of orientational and displacement characters , as in (1).
| (1) |
If the BLs are all equivalent, or if their nature, orientation (and/or displacement) is fixed or otherwise obvious, the letters ![]() may be omitted and only their subscripts used as orientational and displacement characters. These are generally written in a two-line form with orientation characters in the upper and displacement characters in the lower line. In this case, symbol (1) becomes
may be omitted and only their subscripts used as orientational and displacement characters. These are generally written in a two-line form with orientation characters in the upper and displacement characters in the lower line. In this case, symbol (1) becomes
| (2) |
If the orientations (displacements) are all alike, or if they follow unambiguously from displacements (orientations), the corresponding characters may be omitted. In such a case, sequence (1) may be reduced to
| (3) |
An analogous reduction is also possible for sequence (2):
| (4) |
Characters which are redundant may or may not be omitted, and accordingly we speak of non-redundant and redundant symbols , respectively. A symbol may also contain additional marks (dots, primers, etc .) for facilitating the recognition of relevant crystal-chemical features. The smallest repeating part of the periodic sequence of characters, placed between vertical bars (![]() ) indicates periodic polytypes. The number of BL-repeating units per symbol-repeating unit is represented by the corresponding subscript number.
) indicates periodic polytypes. The number of BL-repeating units per symbol-repeating unit is represented by the corresponding subscript number.
When a polytypic structure consists of equivalent BLs and any BL may occupy only one of two alternative positions relative to the preceding BL, the polytypic symbol is reduced to the simplest form, i.e. a sequence of characters of two kinds, e.g. + and - in Häggs's (1943) symbolism, which in turn is simplified to the short-hand form of Zhdanov's (1945) symbolism, indicating the numbers m and n of the consecutive characters + and -, respectively.
Examples of recommended descriptive symbols are given in Appendix 2. Additional details may be found in Dornberger-Schiff, Durovic & Zvyagin (1982).
It is a pleasure to thank A. J. C. Wilson for improving this report by his critical comments.
APPENDIX 1
Examples of the application of the modified Gard nomenclature to some representative mineral groups, followed by traditional names in parentheses (not all examples are strict polytypes); further examples are given in the 1977 report
- Elements
- graphite-2H (graphite-2H)
- graphite-3R (graphite-3R)
- Sulphides
- sternbergite-Oab2c (sternbergite)
- sternbergite-Oabc (argentopyrite)
- pyrrhotite-Hbbc (troilite)
- pyrrhotite-H2a2a6c (6C-type pyrrhotite)
- pyrrhotite-O2a2b11c (11C-type pyrrhotite)
- pearceite-
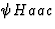 (pearceite)
(pearceite) - pearceite-
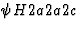 (arsenopolybasite)
(arsenopolybasite) - andorite-Oab12c (andorite)
- andorite-
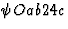 (nakaseite)
(nakaseite) - Halogenides
- atacamite-R2a2a3c (paratacamite)
- Oxides
- taaffeite-4H (taaffeite)
- taaffeite-9R
- gibbsite-
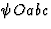 (bayerite)
(bayerite) - gibbsite-Mba2c (hydrargillite)
- Carbonates
- hydrotalcite-2H (manasseite)
- pyroaurite-3R (pyroaurite)
- Borates
- veatchite-Ma2bc (veatchite)
- Sulphates
- coquimbite-3R (paracoquimbite)
- Phosphates, arsenates, and vanadates
- variscite-
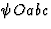 (metavariscite)
(metavariscite) - strengite-
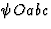 (metastrengite)
(metastrengite) - Silicates
- chloritoid-Aabc (chloritoid-1A)
- zoisite-Mabc (clinozoisite)
- cordierite-1H (indialite)
- anthophyllite-O2abc (anthophyllite)
- wollastonite-Aabc (wollastonite)
- wollastonite-M2abc (parawollastonite)
APPENDIX 2
Examples of descriptive symbols for some polytypic structures, with dependence on choice of BLs, origins and stacking direction
A. Explanatory introduction and explicit table of symbols for two cases(1) ZnS Family. The structures (see Fig. 1) may be considered to consist of BLs chosen as follows (Verma & Krishna, 1966).
| Figure 1: Polytype 15R of ZnS. Structural arrangement in the ( |
 |
(a) Sheets of Zn-S dumb-bells projecting in close-packing positions denoted by A, B, C, with any two successive positions corresponding to different letters. Sequences AB, BC, CA are equivalent and may be considered as displacements q+; the reverse sequences AC, CB, BA give displacements of opposite sense q-.
(b) Sheets of ZnS4 tetrahedra sharing common corners. The displacements of these BLs are characterized by the same sequences as above and also serve as orientational vectors Q+ and Q-. Since the displacement and orientation senses coincide, one kind of character is sufficient for the description.
An example is given in Table 1.
| Substance | BLs chosen | Full symbol (redundant) | Simplified symbol (nonredundant) |
Number of BLs per repeat |
Space group |
| ZnS (15R) |
(a) | |q+q+q+q-q-| 3 | |+++-|3 | 15 | R3m |
| (b) | |Q+q+Q+q+Q+q +Q-q-Q-q-| 3 |
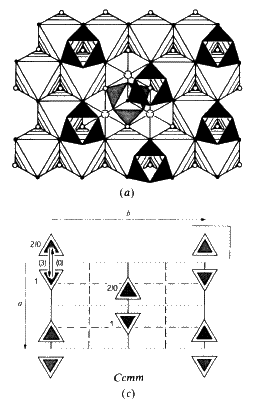
(2) Strontium germanate : SrGeO3. Sheets of SrO6 octahedra (sharing edges, see Fig. 2) are linked by sheets of isolated three-membered rings of GeO4 tetrahedra: all rings share their non-bridging O atoms with octahedra and project onto every third triangle between the bases of adjacent SrO6 octahedra (Dornberger-Schiff, 1961). There are three choices of BLs.
| Figure 2: Polytypism in SrGeO3. (a) General structural arrangement viewed down [001]. Sheet of SrO6 octahedra and upper sheet of Ge3O9 rings (heavily dotted - almost black - triangles). Three octahedra in the central part are left open to show how the rings of the lower tetrahedral sheet (lightly dotted triangles) are attached to the octahedral sheet. (b) Projection along [100] with the three choices of `building layers', BL(a), BL(b), and BL(c) indicated by braces. The origins for BL(a) and BL(b) are chosen in the centres of the germanate rings at points with site symmetries |
|
 |
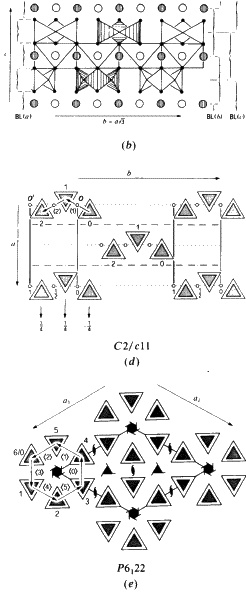 |
(a) Two kinds of BLs, with BL(1) an octahedral sheet and BL(2) a sheet of isolated tetrahedral rings. Non-bridging oxygen ring atoms belong half to one, half to the adjacent BL. Since successive BLs of one kind regularly alternate with respect to their orientations, displacements (between ring centres) only are sufficient to describe the polytypes.
(b) One kind of equivalent BL: germanate sheets with planes of Sr half-atoms attached on either side. Displacements as in (a) are used.
(c) One kind of equivalent BL: octahedral sheets with planes of Ge and bridging O half-atoms attached to them on either side. Displacement vectors as in (a) and (b) are to be considered orientational vectors. These vectors have length a/3 in the normal projection on the orthohexagonal basis plane ab and are related by counterclockwise rotations of ![]() about the c axis. They are accordingly assigned the characters n = 0, 1,
about the c axis. They are accordingly assigned the characters n = 0, 1, ![]() , 5. The character 0 (or 6) denotes the vector parallel to
, 5. The character 0 (or 6) denotes the vector parallel to ![]() of the orthogonal C-centered base
of the orthogonal C-centered base ![]() .
.
An example is given in Table 2.
| Substance SrGeO3 |
BLs chosen | Full symbol (redundant) | Simplified symbol (nonredundant) |
Number of equivalent BLs per repeat |
Space group |
| (2O) | (a)(b) | |p3p0| | |30| | 2 | Ccmm |
| (c) | |P3P0| | ||||
| (2M) | (a)(b) | |p1p2| | |12| | 2 | C2/c11 |
| (c) | |P1P2| | ||||
| (6H) | (a)(b) | |p3p4p5p 0p1p2| | |345012| | 6 | P6122 |
| (c) | |P3P4P5P 0P1P2| |
B. Illustrative papers on polytypic substances with description of symbolism used
- Astrophyllite
- Zvyagin & Vrublevskaya (1976).
- Kaolinite-type structures
- Zvyagin (1964, 1967) (tri- and di-octahedral polytypes).
- Dornberger-Schiff & Durovic (1975) (tri-, di- and mono-octahedral polytypes).
- Mica
- Zvyagin (1964, 1967) (tri-octahedral polytypes).
- Takeda (1967) (tri-octahedral polytypes).
- Dornberger-Schiff, Backhaus & Durovic (1982) (tri-, di and mono-octahedral polytypes).
- Vermiculites
- Weiss & Durovic (1980).
References
Dornberger-Schiff, K. (1961). Kristallografiya, 6, 859-868; Sov. Phys. Crystallogr. (1962), 6, 694-703.
Dornberger-Schiff, K., Backhaus, K. O. & Durovic, S. (1982). Clays Clay Miner. 30, 364-374.
Dornberger-Schiff, K. & Durovic, S. (1974). Collected Abstracts, 2nd European Crystallographic Meeting, Keszthely.
Dornberger-Schiff, K, & Durovic, S. (1975). Clays Clay Miner. 23, 219-246.
Dornberger-Schiff, K, Durovic, S. & Zvyagin, B. B. (1982). Cryst. Res. Tech. 17, 1449-1457.
Gard, J. A. (1966). Nature (London) 211, 1078-1079.
Hägg, G. (1943). Ark Kem. Mineral. Geol. 16B, 1-6.
International Tables for Crystallography (1983). Vol. A. Dordrecht: Reidel.
Ramsdell, L. S. (1947). Am. Mineral. 32, 64-82.
Strunz, H. (1966). Mineralogische Tabellen, Vol. 4. Leipzig: Academischer Verlag.
Takeda, H. (1967). Acta Cryst. 22, 845-853.
Verma, A. R. & Krishna, P. (1966). Polymorphism and Polytypism in Crystals, pp. 83 ff. New York: Wiley.
Weiss, Z. & Durovic, S. (1980). Acta Cryst. A36, 633-640.
Zhdanov, G. S. (1945). C. R. (Dokl.) Acad. Sci. URSS, 48, 40-43.
Zvyagin, B. B. (1964). Elektronografiya i Strukturnaya Kristallografiya Glinistykh Mineralov. Moskva: Nauka.
Zvyagin, B. B. (1967). Electron Diffraction Analysis of Clay Mineral Structures. New York: Plenum Press.
Zvyagin, B. B. (1974). Collected Abstracts, 2nd European Crystallographic Meeting, Keszthely.
Zvyagin, B. B. & Vrublevskaya, Z. V. (1976). Kristallografiya, 21, 949-954; Sov. Phys. Crystallogr. (1976), 21, 542-545.
-
...compound:*
- *The Ad-Hoc Committee is aware that this notation does not conform with that of International Tables for Crystallography (1983) or Structure Reports but recommends its retention since it is embedded in the literature. It is, however, recommended that use of these symbols be confined exclusively to polytype description terminology.
These pages are maintained by the Commission Last updated: 15 Oct 2021

